2.2 — Short Run and Long Run
ECON 306 • Microeconomic Analysis • Spring 2022
Ryan Safner
Assistant Professor of Economics
safner@hood.edu
ryansafner/microS22
microS22.classes.ryansafner.com
The “Runs” of Production
“Time”-frame usefully divided between short vs. long run analysis
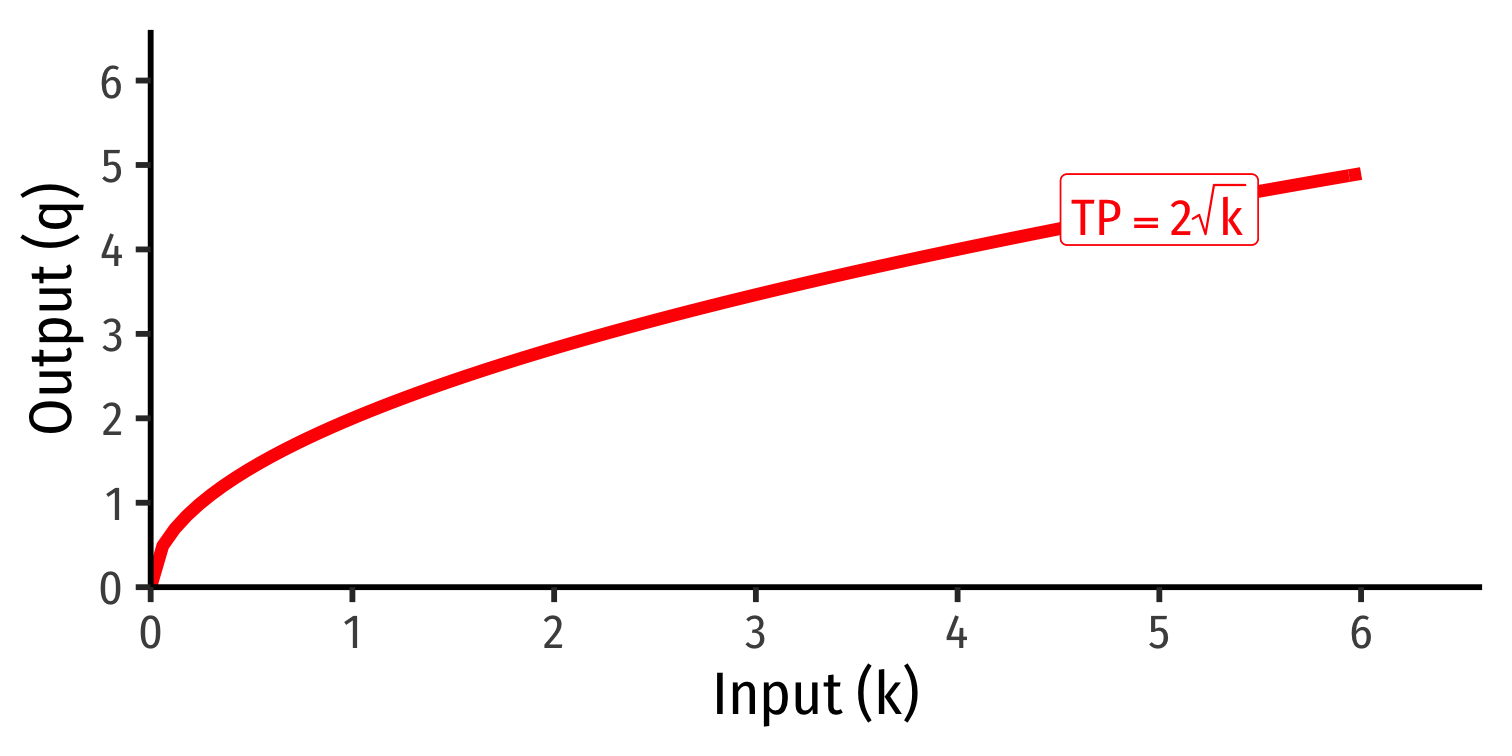
Short run: at least one factor of production is fixed (too costly to change) q=f(¯k,l)
- Assume capital is fixed (i.e. number of factories, storefronts, etc)
- Short-run decisions only about using labor

The “Runs” of Production
“Time”-frame usefully divided between short vs. long run analysis
Long run: all factors of production are variable (can be changed) q=f(k,l)

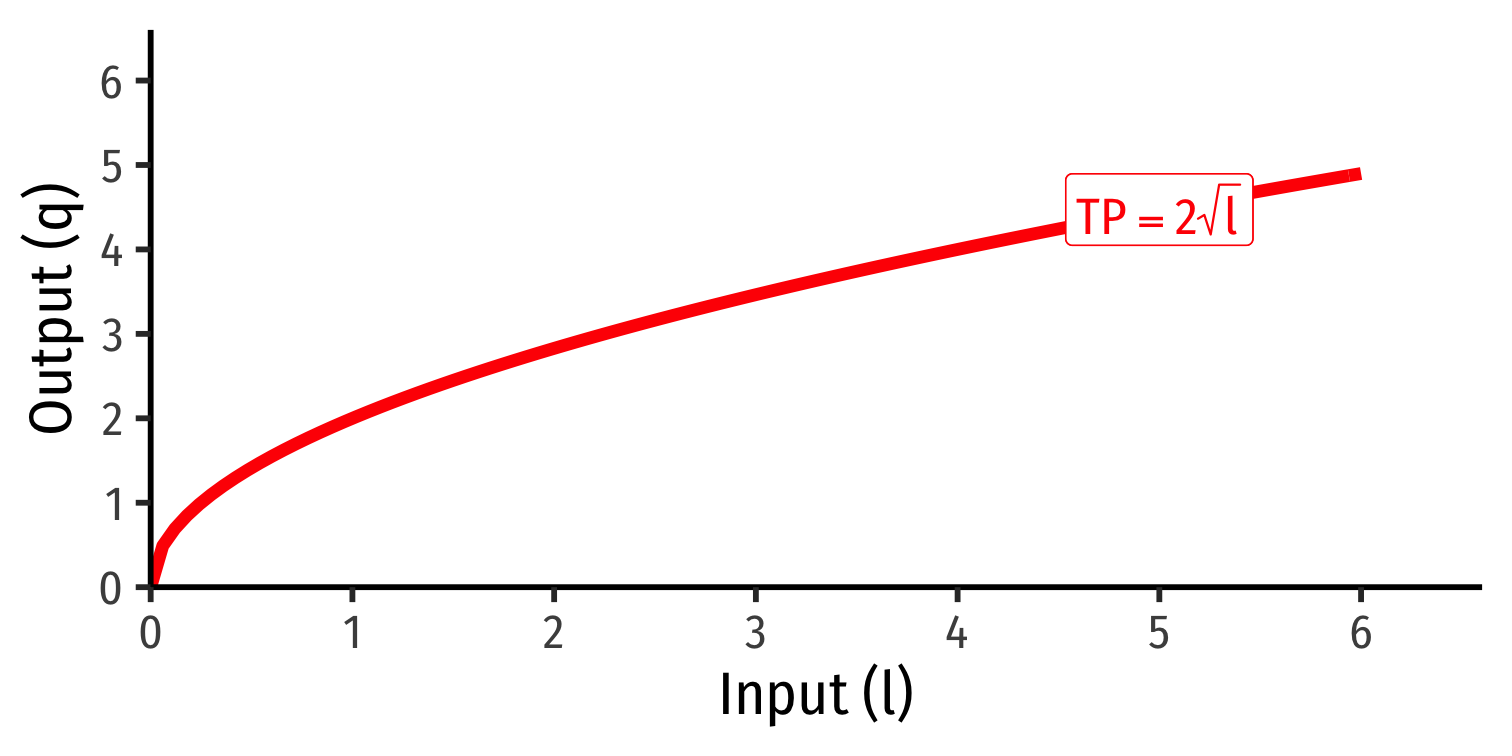
Production in the Short Run
Production in the Short Run: Example
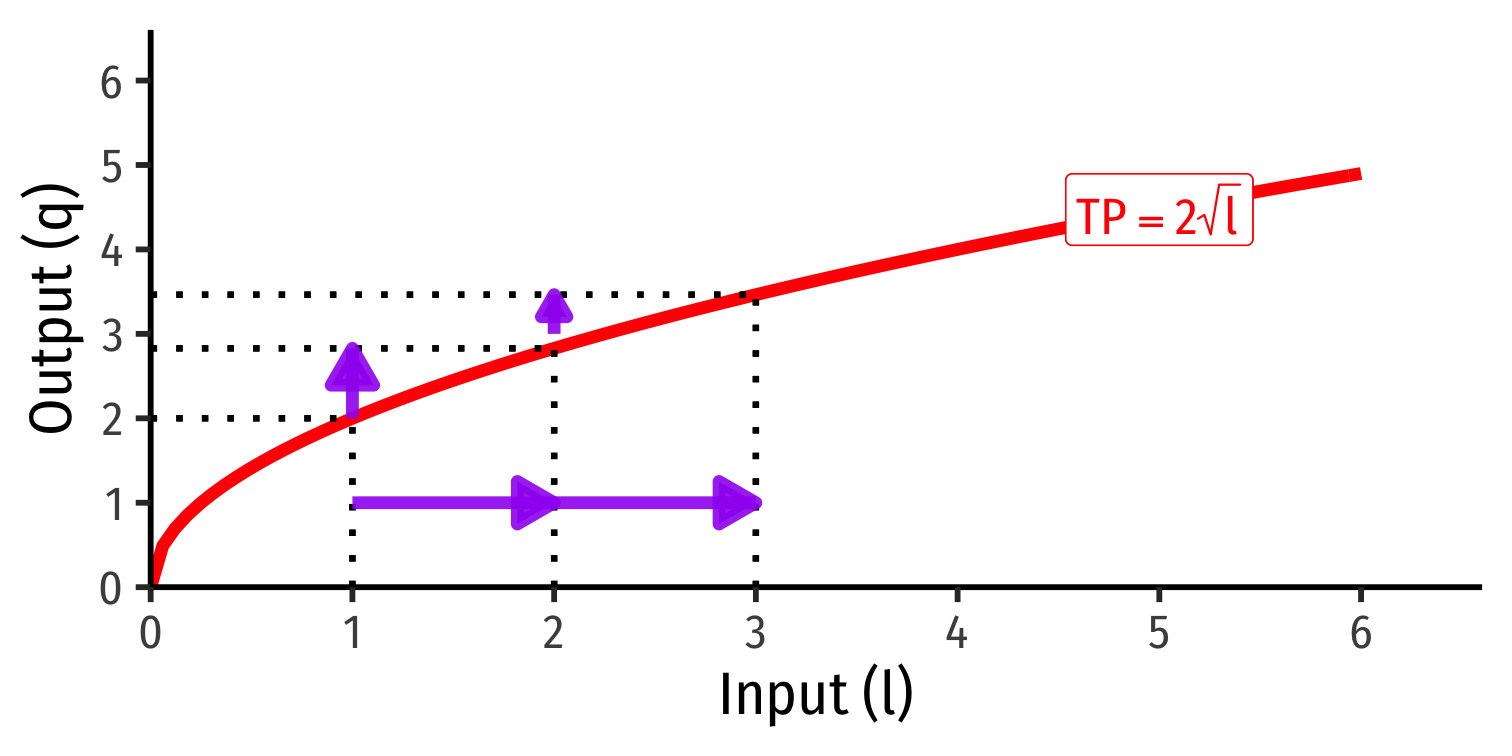
Example: Consider a firm with the production function q=k0.5l0.5
- Suppose in the short run, the firm has 4 units of capital.
- Derive the short run production function.
- What is the total product (output) that can be made with 4 workers?
- What is the total product (output) that can be made with 5 workers?
Production in the Short Run: Example
Example: Consider a firm with the production function q=k0.5l0.5
- Suppose in the short run, the firm has 4 units of capital.
- Derive the short run production function.
- What is the total product (output) that can be made with 4 workers?
- What is the total product (output) that can be made with 5 workers?

Marginal Products
The marginal product of an input is the additional output produced by one more unit of that input (holding all other inputs constant)
Like marginal utility
Similar to marginal utilities, I will give you the marginal product equations

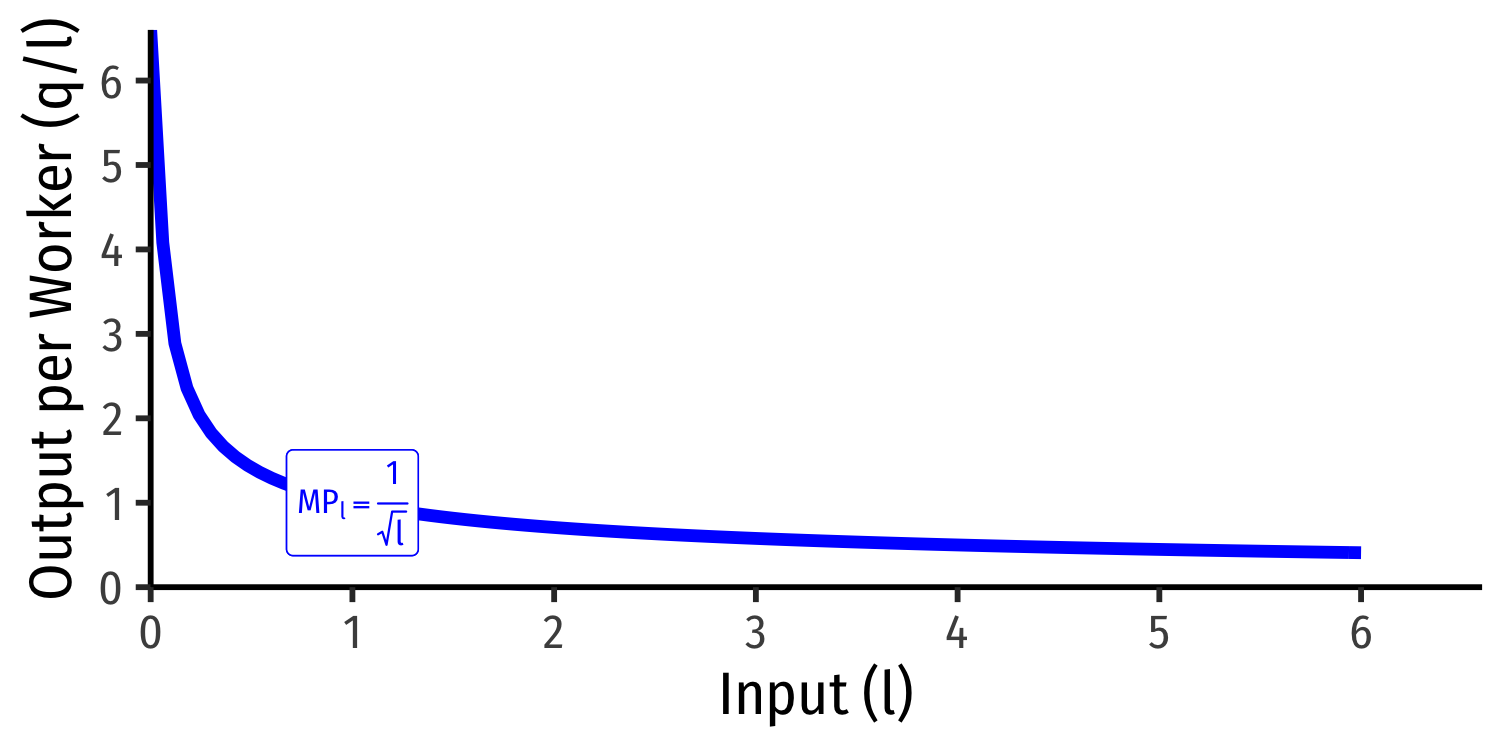
Marginal Product of Labor
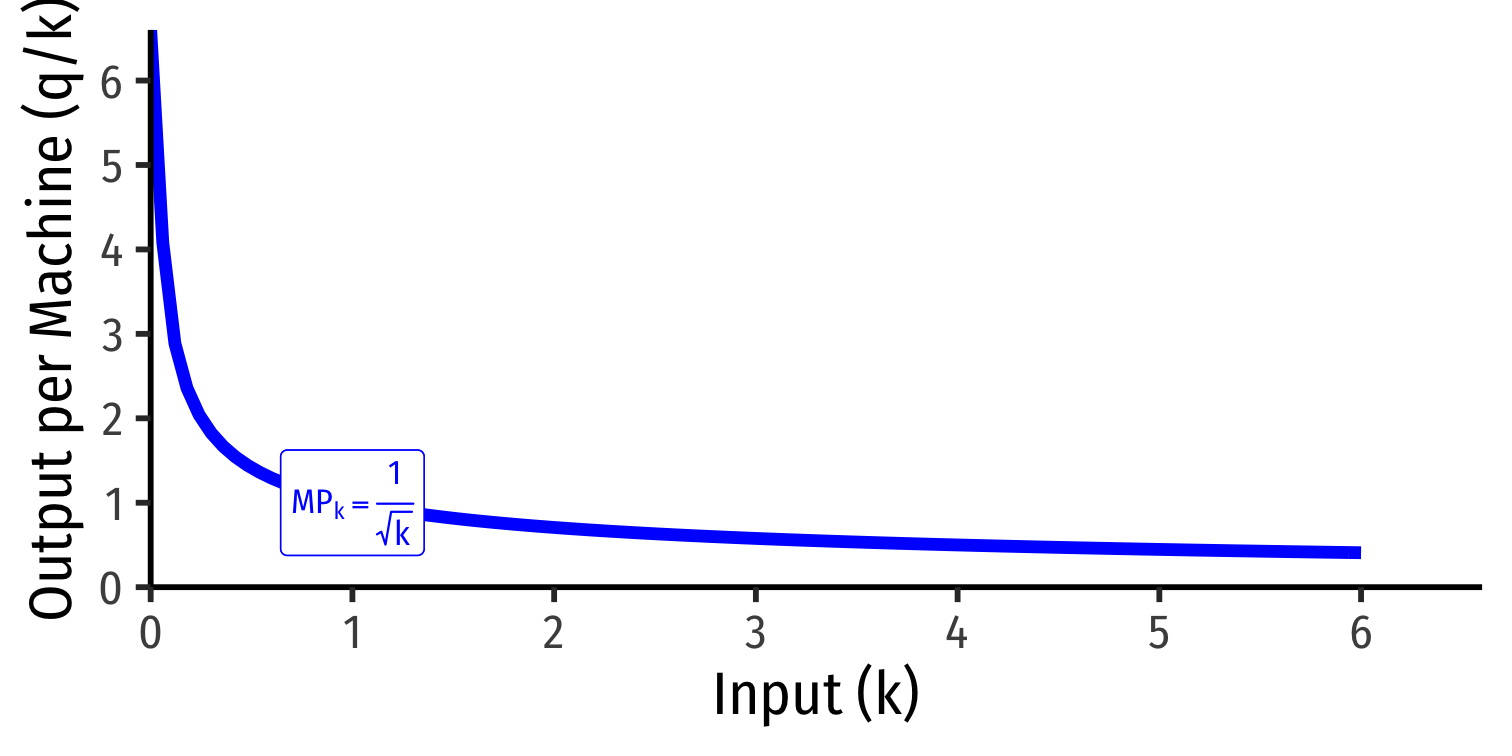
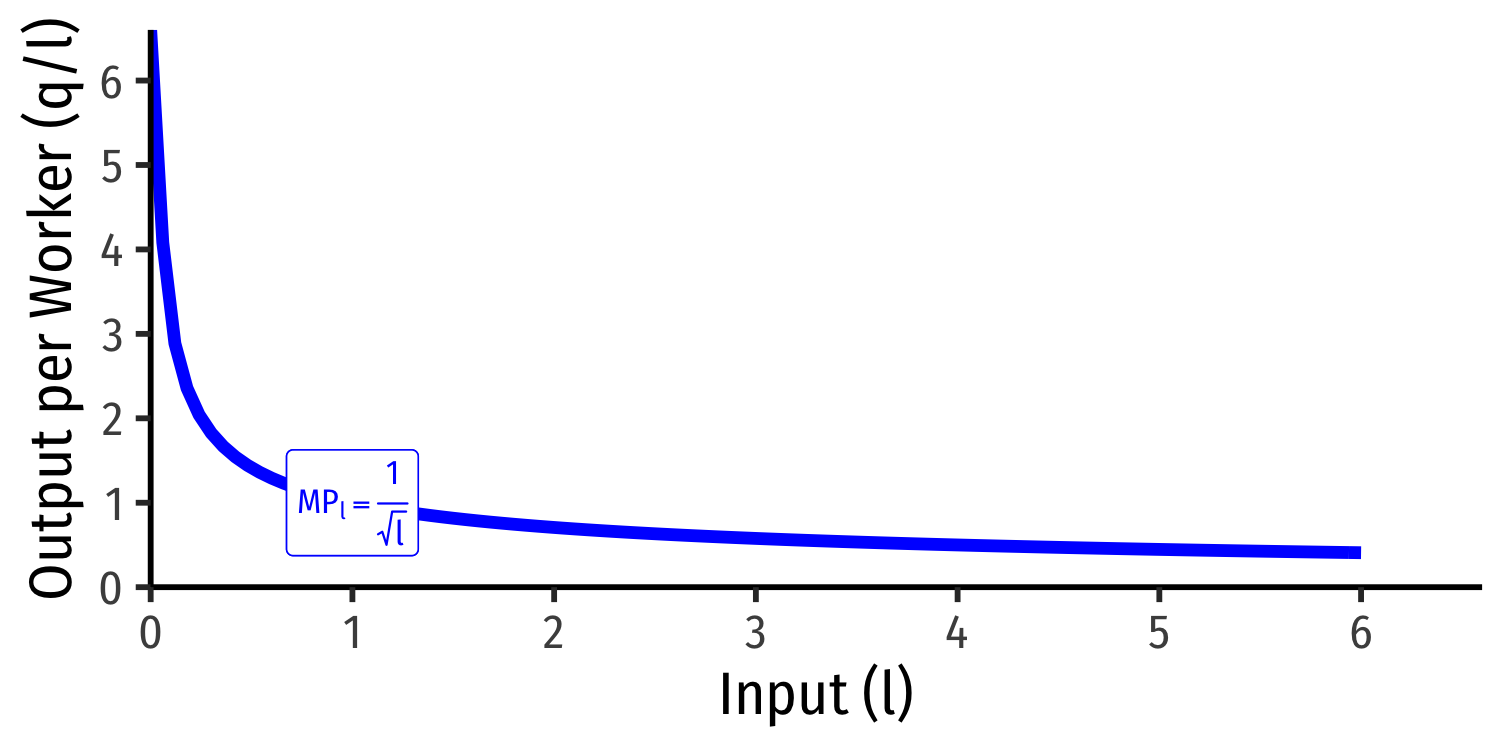
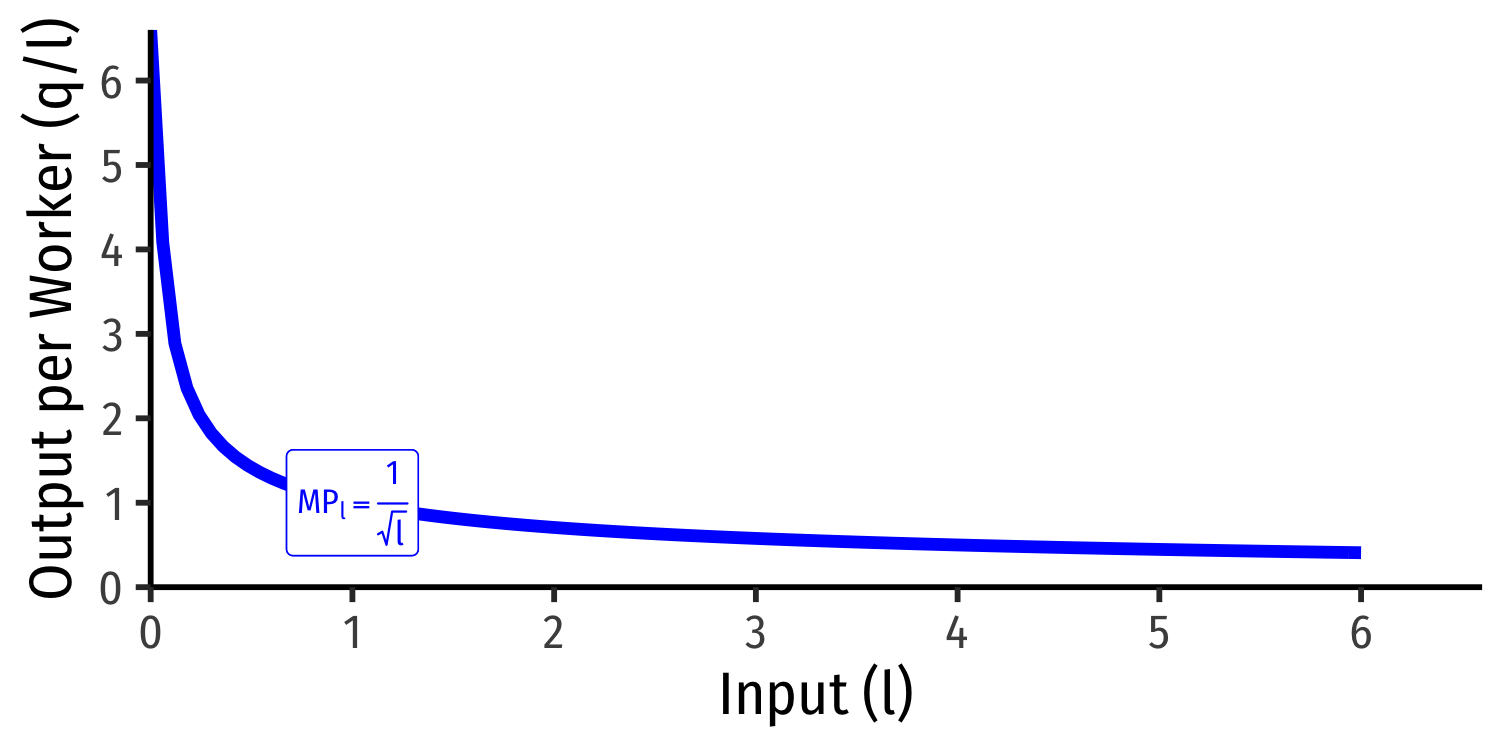
Marginal product of labor (MPl): additional output produced by adding one more unit of labor (holding k constant) MPl=ΔqΔl
MPl is slope of TP at each value of l!
- Note: via calculus: ∂q∂l
Marginal Product of Capital
Marginal product of capital (MPk): additional output produced by adding one more unit of capital (holding l constant) MPk=ΔqΔk
MPk is slope of TP at each value of k!
- Note: via calculus: ∂q∂k
Note we don't consider capital in the short run!
Diminishing Returns
Law of Diminishing Returns: adding more of one factor of production holding all others constant will result in successively lower increases in output
In order to increase output, firm will need to increase all factors!
Diminishing Returns
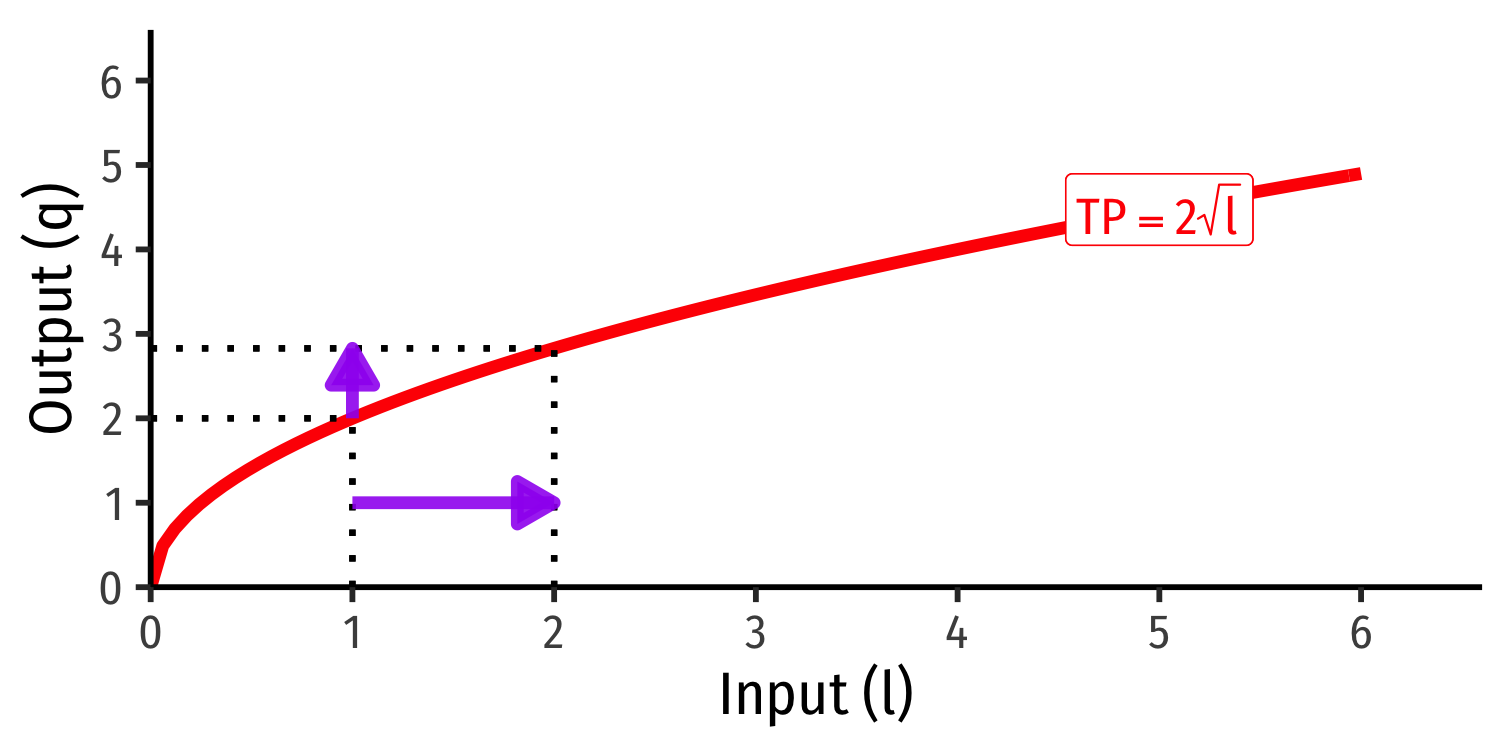
Law of Diminishing Returns: adding more of one factor of production holding all others constant will result in successively lower increases in output
In order to increase output, firm will need to increase all factors!

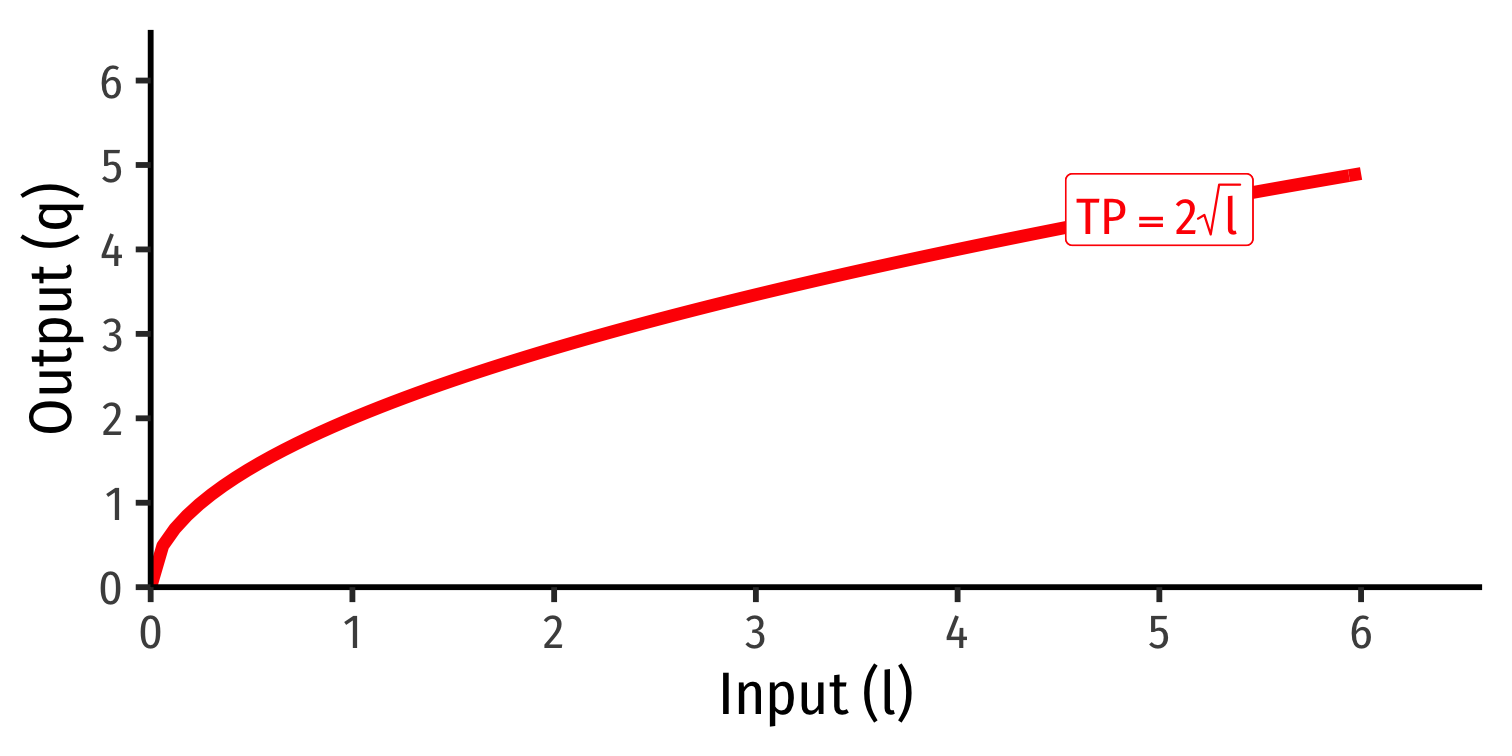
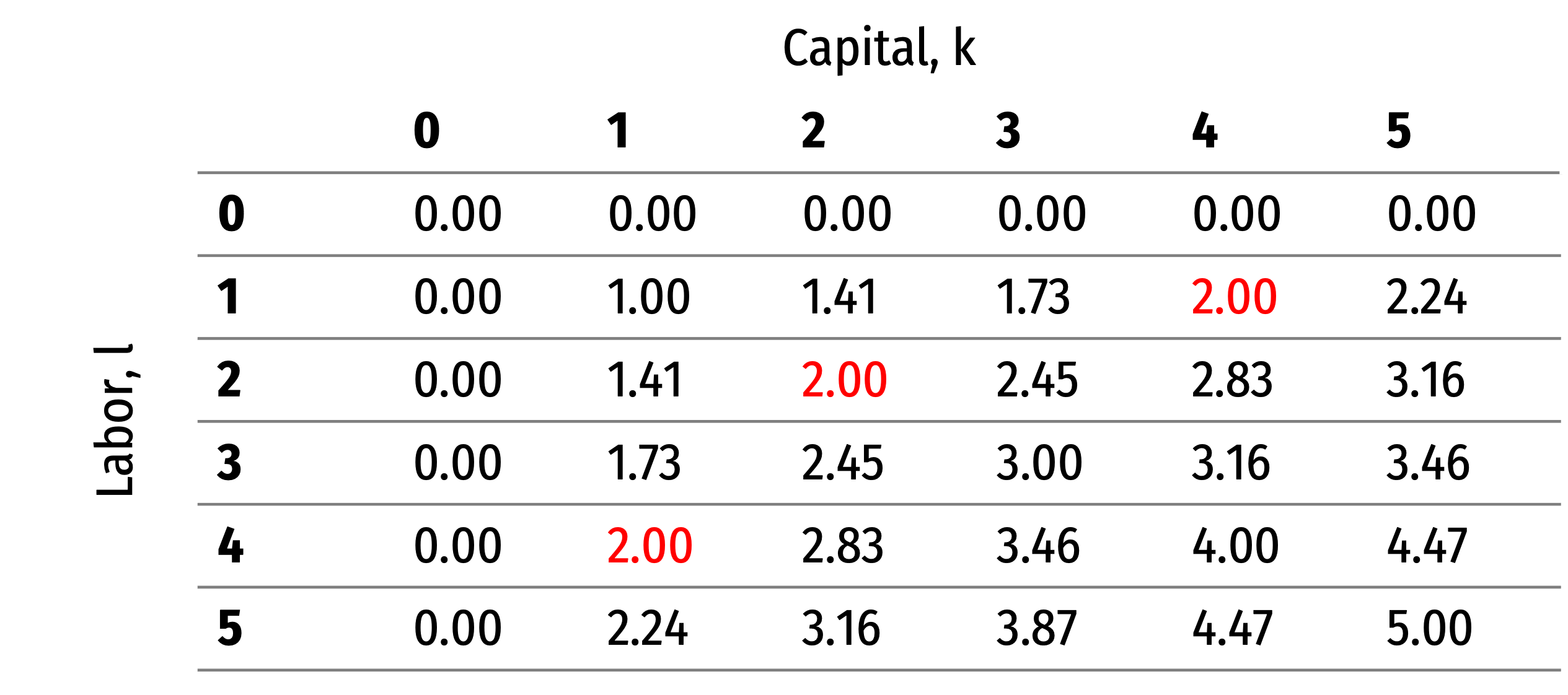
Production Functions and Marginal Product
- A quick trick to roughly† estimate MPl
MPl≈q2−q1l2−l1
| l | q | MPl |
|---|---|---|
| 0 | 0.00 | − |
| 1 | 2.00 | 2.00−0.00=2.00 |
| 2 | 2.83 | 2.83−2.00=0.83 |
| 3 | 3.46 | 3.46−2.83=0.63 |
† Note these are approximate. Technically, MPl is defined via calculus as an infinitesimal change in l, whereas these are discrete changes.
Average Product of Labor (and Capital)
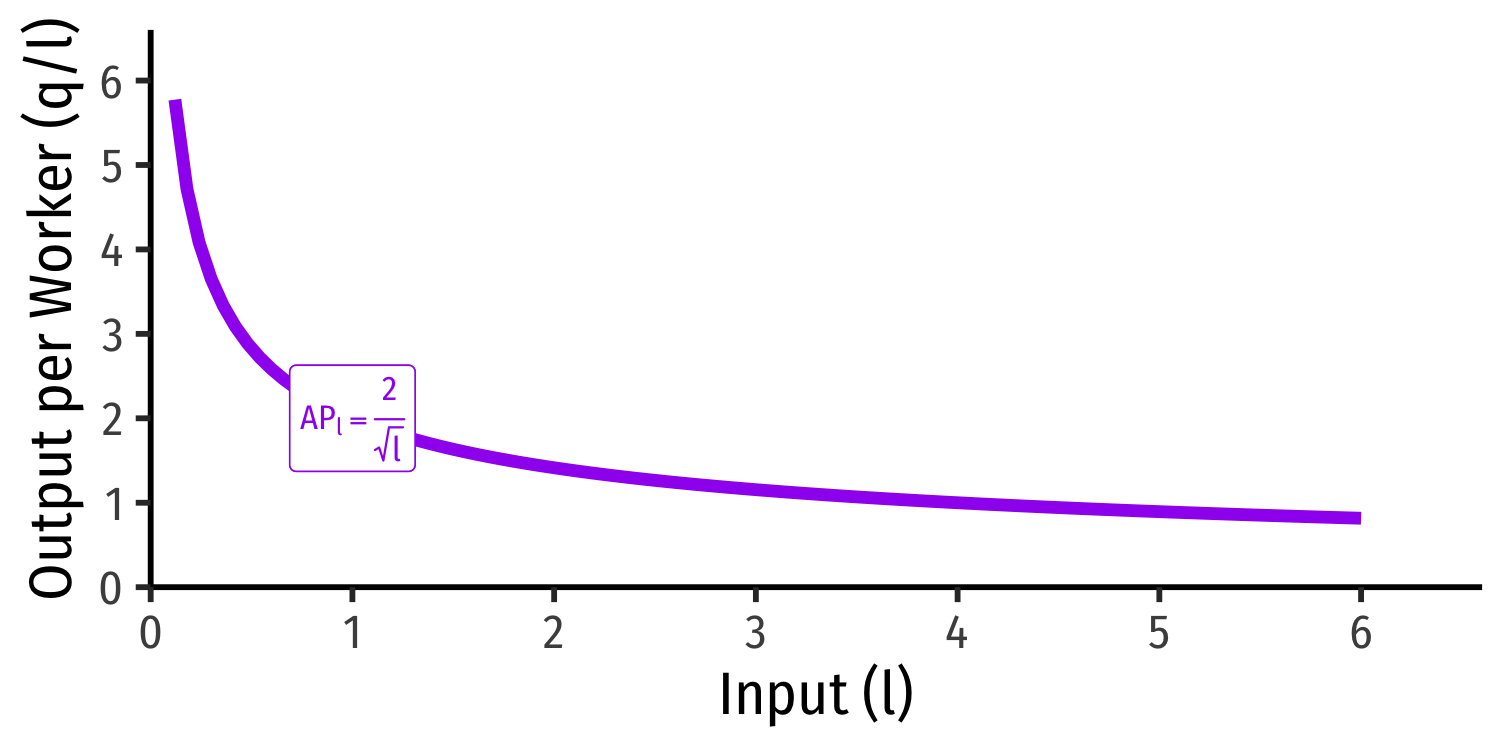
Average product of labor (APl): total output per worker APl=ql
A measure of labor productivity
Average product of capital (APk): total output per unit of capital APk=qk
Production in the Short Run: Example II
Example: Suppose a firm has the following production function: q=2k+l2
- Suppose in the short run, the firm has 10 units of capital.
- Write an equation for the short run production function.
- Calculate the total product(s), marginal product(s), and average product(s) for each of the first 3 workers.
The Firm's Problem: Long Run
The Long Run
- In the long run, all factors of production are variable
q=f(k,l)
Can build more factories, open more storefronts, rent more space, invest in machines, etc.
So the firm can choose both l and k
The Firm's Problem
Based on what we've discussed, we can fill in a constrained optimization model for the firm
- But don't write this one down just yet!
The firm's problem is:
Choose: < inputs and output >
In order to maximize: < profits >
Subject to: < technology >
- It's actually much easier to break this into 2 stages. See today’s class notes page for an example using only one stage.

The Firm's Two Problems
1st Stage: firm's profit maximization problem:
Choose: < output >
In order to maximize: < profits >
- We'll cover this later...first we'll explore:

The Firm's Two Problems
1st Stage: firm's profit maximization problem:
Choose: < output >
In order to maximize: < profits >
- We'll cover this later...first we'll explore:
2nd Stage: firm's cost minimization problem:
Choose: < inputs >
In order to minimize: < cost >
Subject to: < producing the optimal output >
- Minimizing costs ⟺ maximizing profits

Long Run Production
Example: q=√lk
Many input-combinations yield the same output!
So how does the firm choose the optimal combination?
Isoquants and MRTS
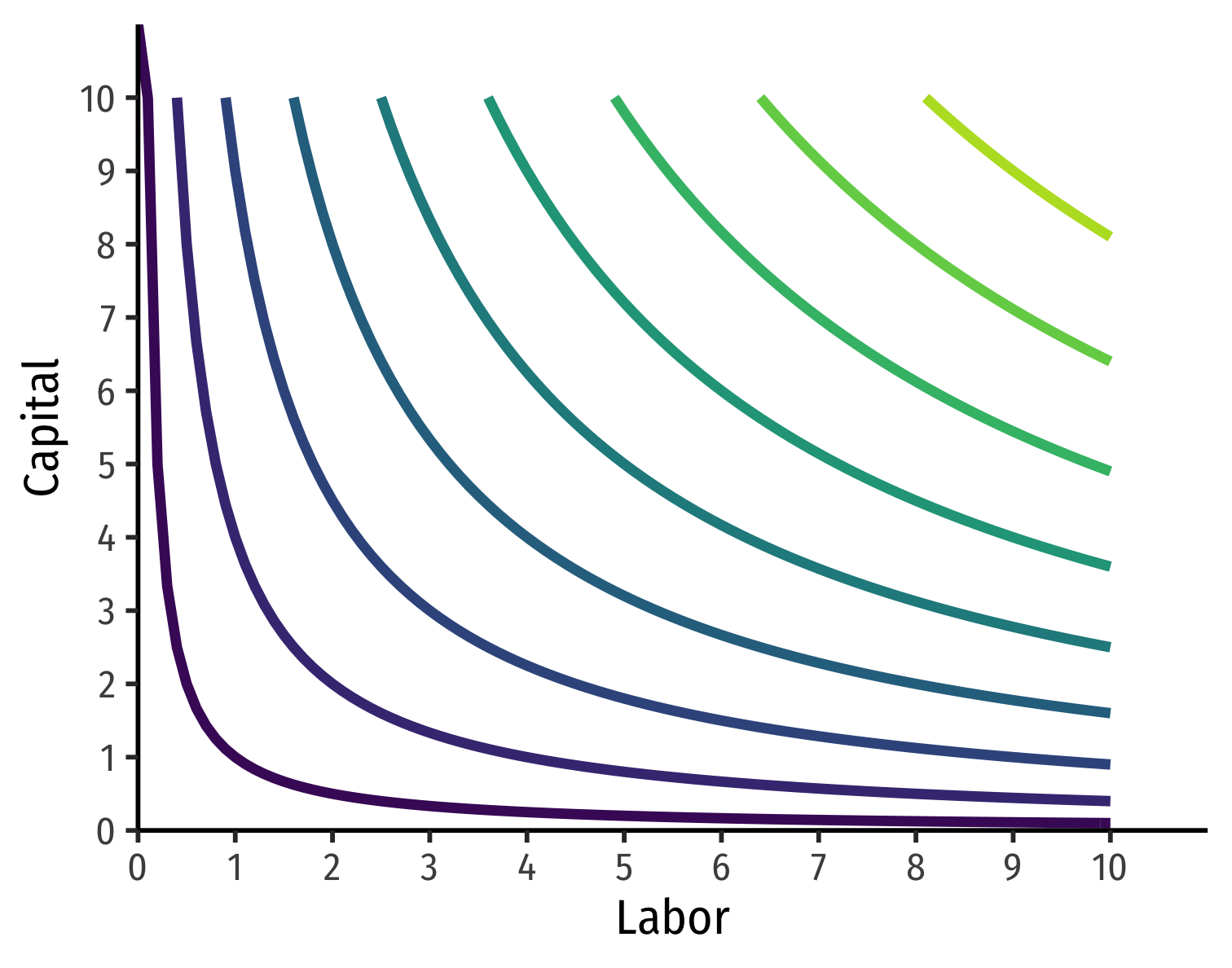
Isoquant Curves
- We can draw an isoquant indicating all combinations of l and k that yield the same q

Isoquant Curves
We can draw an isoquant indicating all combinations of l and k that yield the same q
Combinations above curve yield more output; on a higher curve
- D>A=B=C
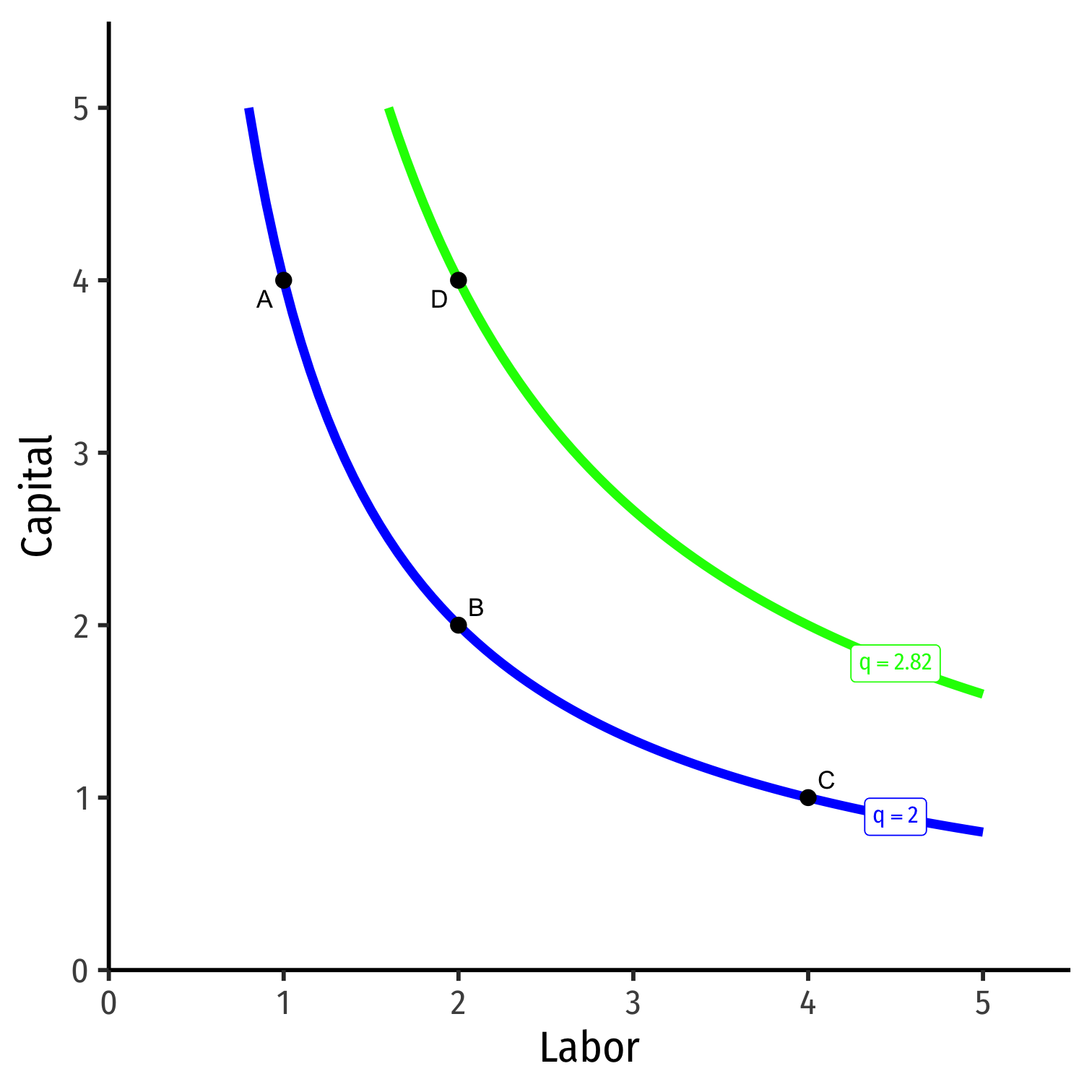
Isoquant Curves
We can draw an isoquant indicating all combinations of l and k that yield the same q
Combinations above curve yield more output; on a higher curve
- D>A=B=C
Combinations below the curve yield less output; on a lower curve
- E<A=B=C

Marginal Rate of Technical Substitution I
- If your firm uses fewer workers, how much more capital would it need to produce the same amount?

Marginal Rate of Technical Substitution I
If your firm uses fewer workers, how much more capital would it need to produce the same amount?
Marginal Rate of Technical Substitution (MRTS): rate at which firm trades off one input for another to yield same output
Firm's relative value of using l in production based on its tech:
“We could give up (MRTS) units of k to use 1 more unit of l to produce the same output.”

Marginal Rate of Technical Substitution II

Marginal Rate of Technical Substitution II
MRTS is the slope of the isoquant MRTSl,k=−ΔkΔl=riserun
Amount of k given up for 1 more l
Note: slope (MRTS) changes along the curve!
Law of diminishing returns!
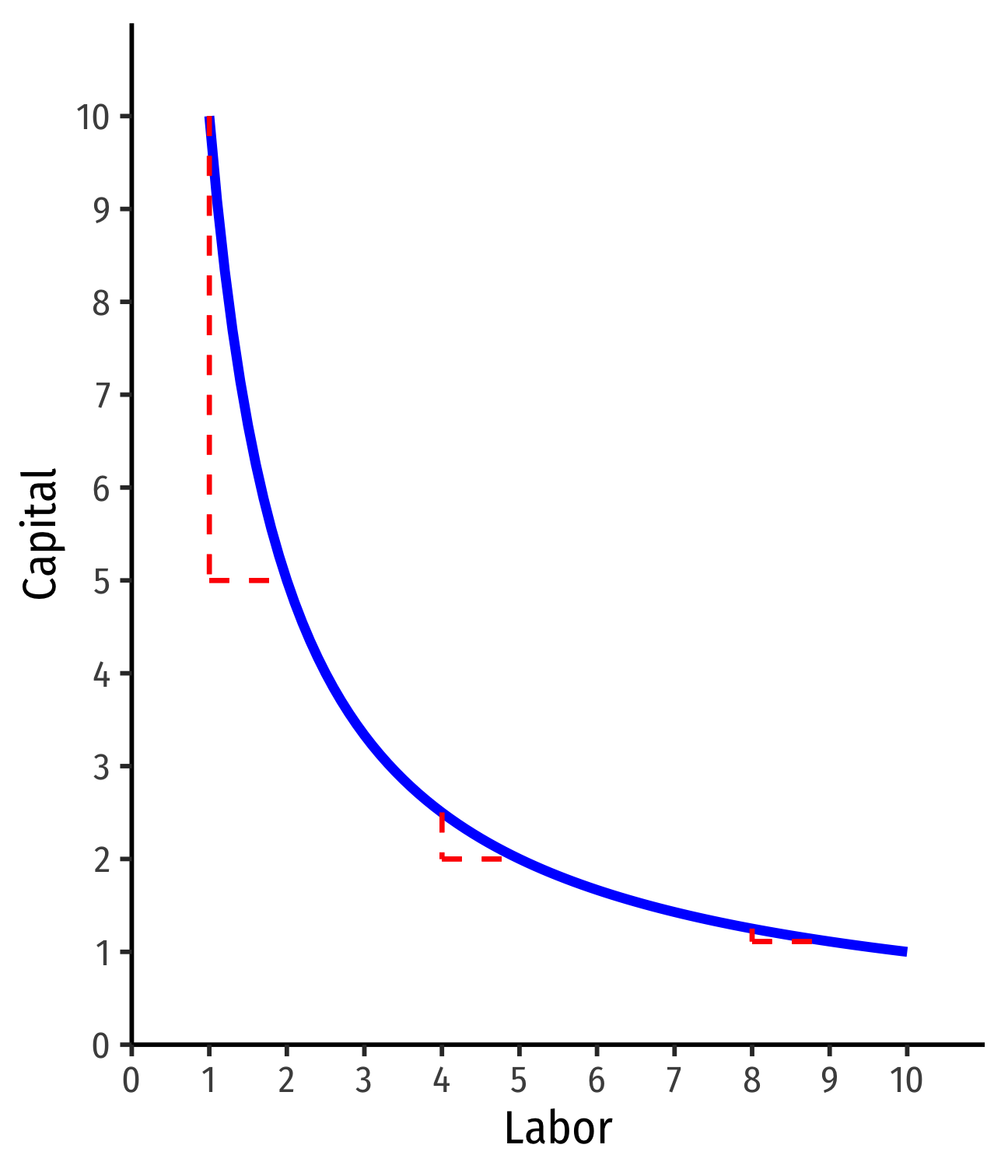
MRTS and Marginal Products
- Relationship between MP and MRTS:
ΔkΔlMRTS=−MPlMPk
See proof in today's class notes
Sound familiar? 🧐
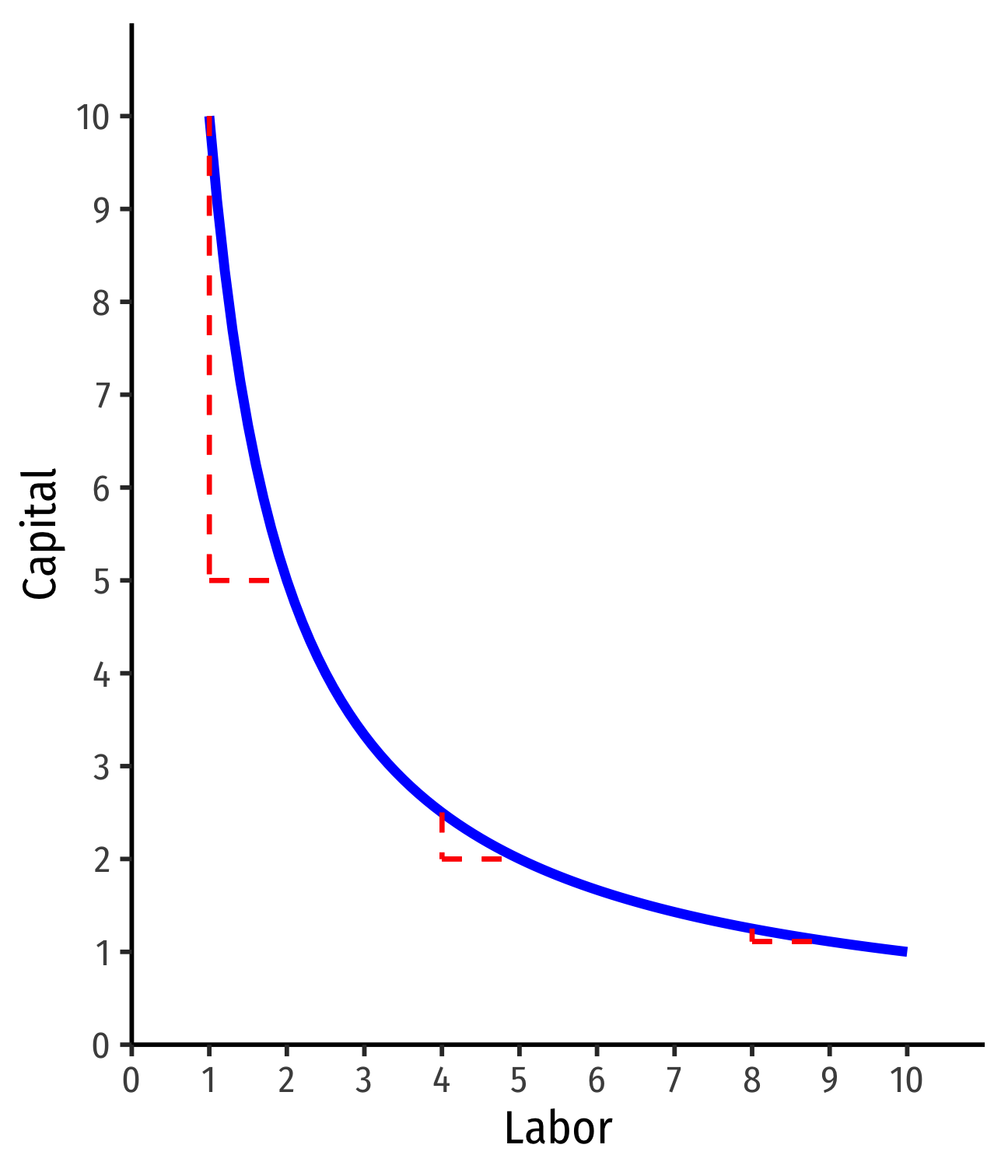
Special Case I: Perfect Substitutes
Example: Consider Bank Tellers (l) and ATMs (k)
Suppose 1 ATM can do the work of 2 bank tellers
Perfect substitutes: inputs that can be substituted at same fixed rate and yield same output
MRTSl,k=−0.5 (a constant!)
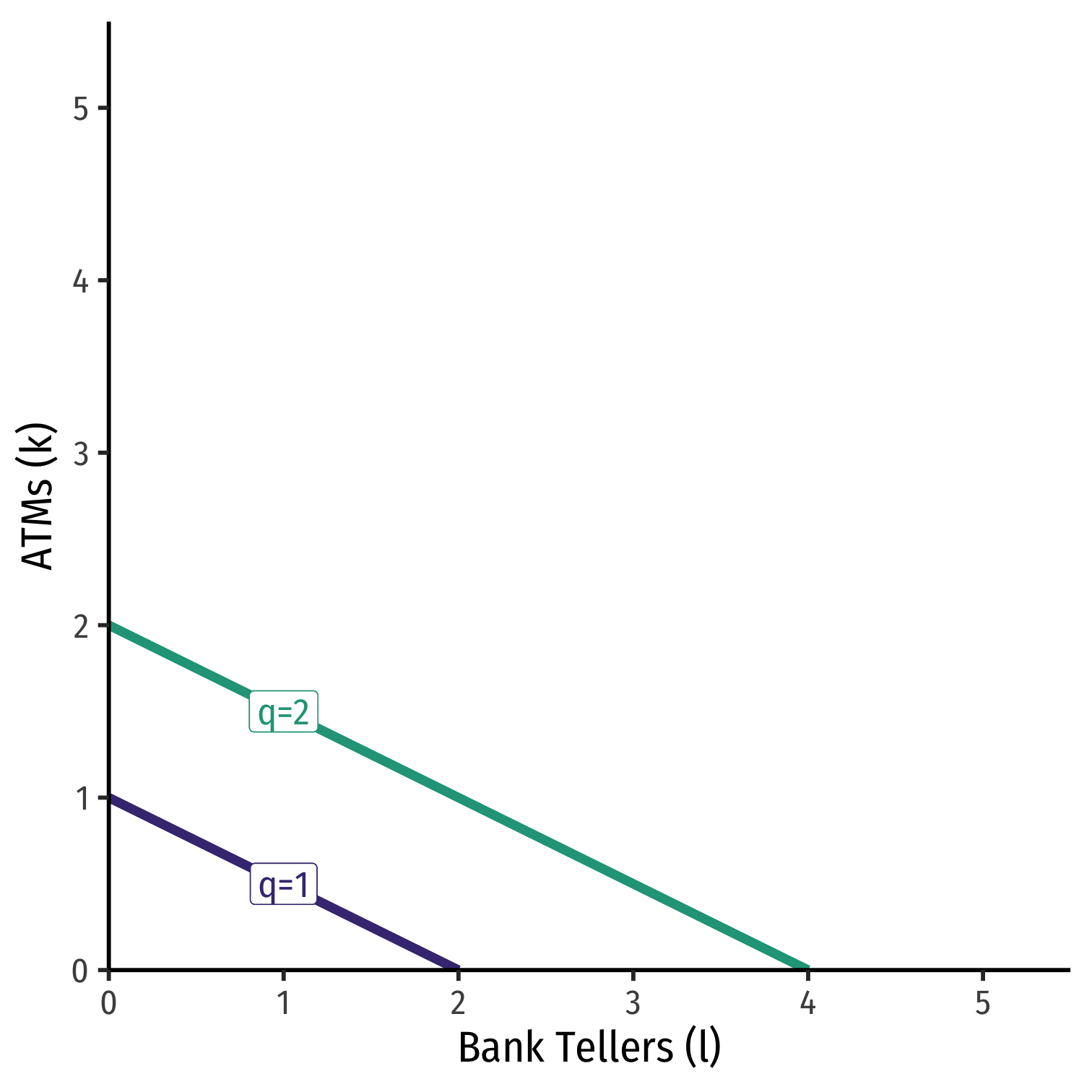
Special Case II: Perfect Complements
Example: Consider buses (k) and bus drivers (l)
Must combine together in fixed proportions (1:1)
Perfect complements: inputs must be used together in same fixed proportion to produce output
MRTSl,k: ?
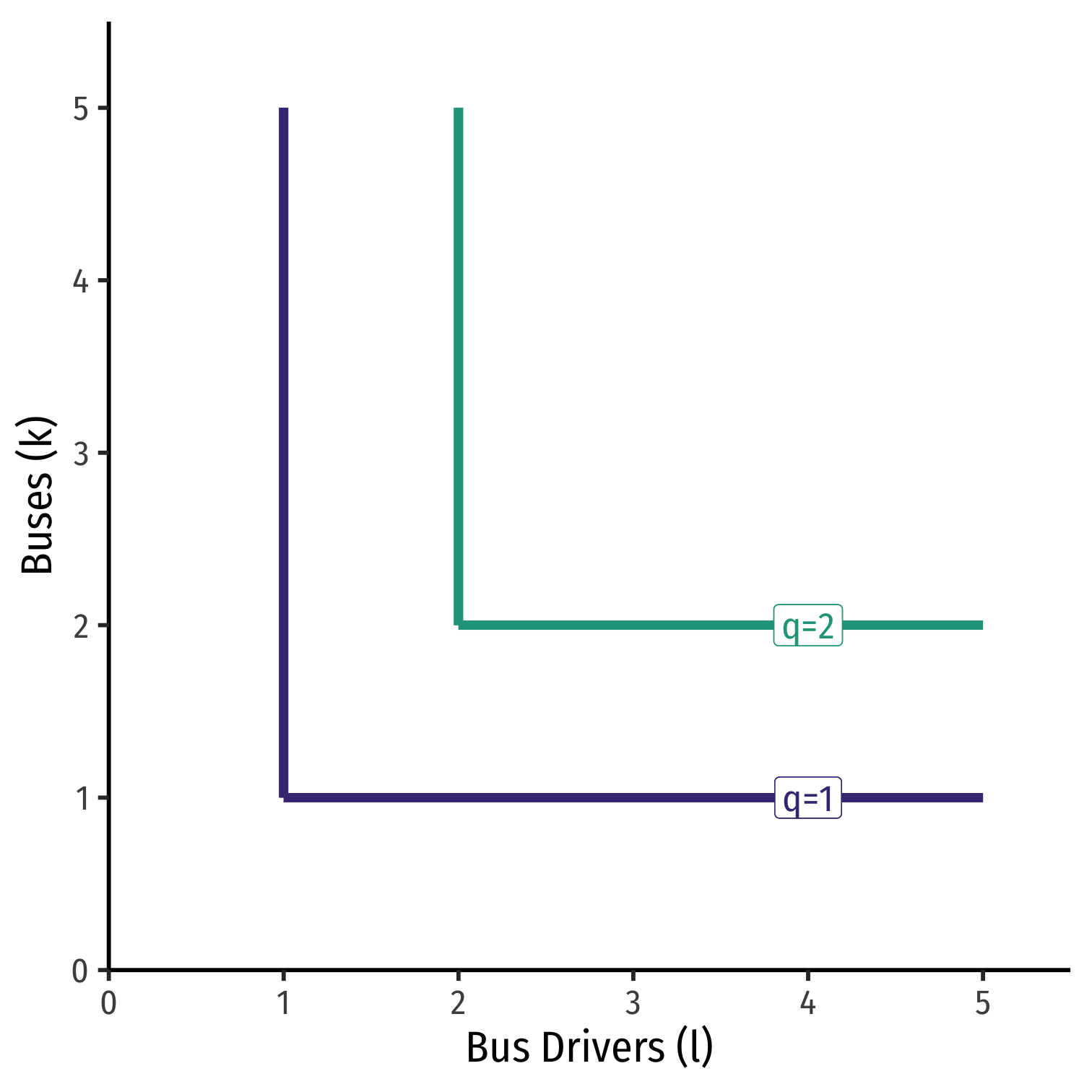
Common Case: Cobb-Douglas Production Functions
- Again: very common functional form in economics is Cobb-Douglas
q=Akalb
Where a,b>0
- often a+b=1
A is total factor productivity
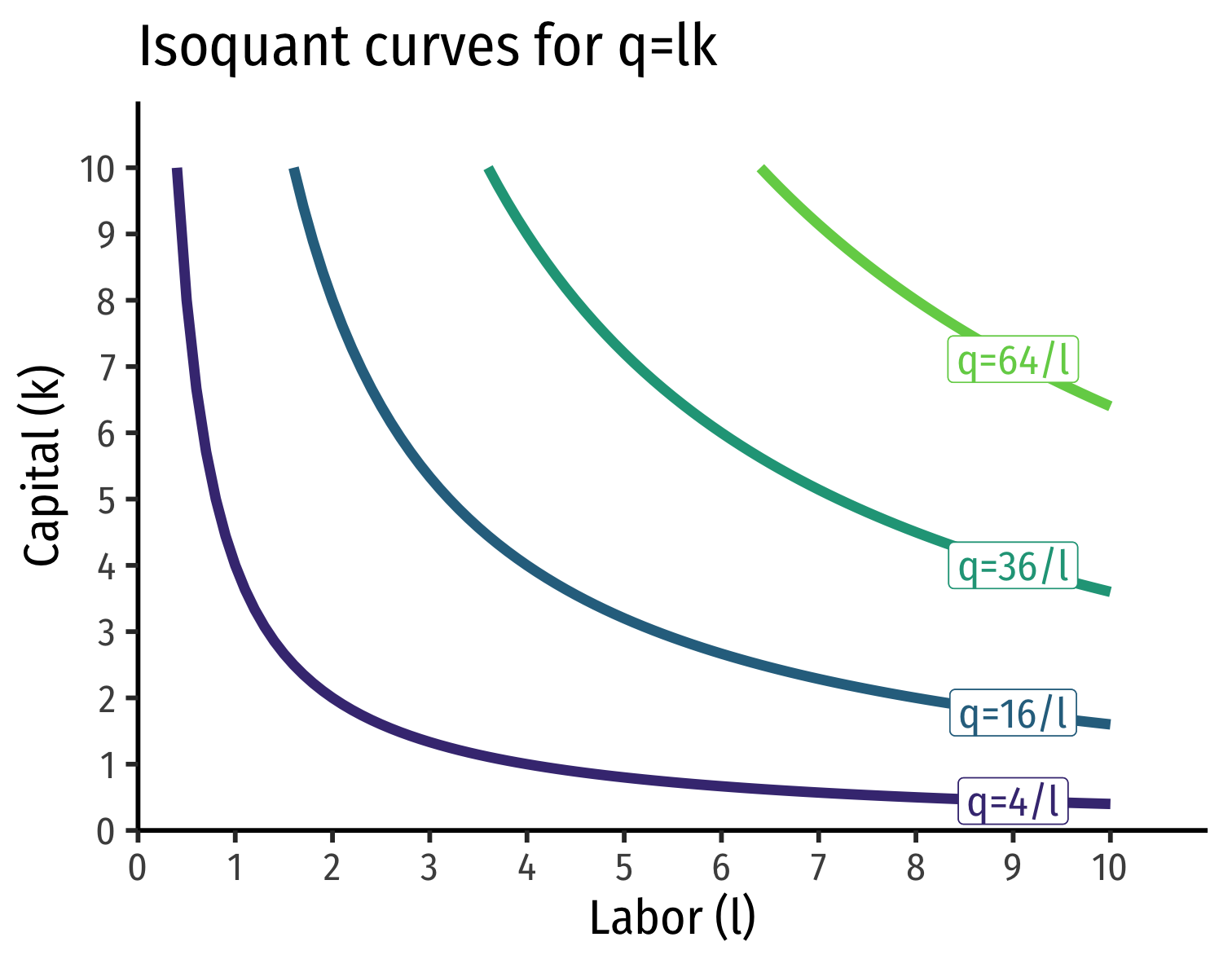
Practice
Example: Suppose a firm has the following production function: q=2lk
Where its marginal products are:
MPl=2kMPk=2l
Put l on the horizontal axis and k on the vertical axis. Write an equation for MRTSl,k.
Would input combinations of (1,4) and (2,2) be on the same isoquant?
Sketch a graph of the isoquant from part 2.
Isocost Lines
Isocost Lines
If your firm can choose among many input combinations to produce q, which combinations are optimal?
Those combination that are cheapest
Denote prices of each input as:
- w: price of labor (wage)
- r: price of capital
Let C be total cost of using inputs (l,k) at market prices (w,r) to produce q units of output:
C(w,r,q)=wl+rk

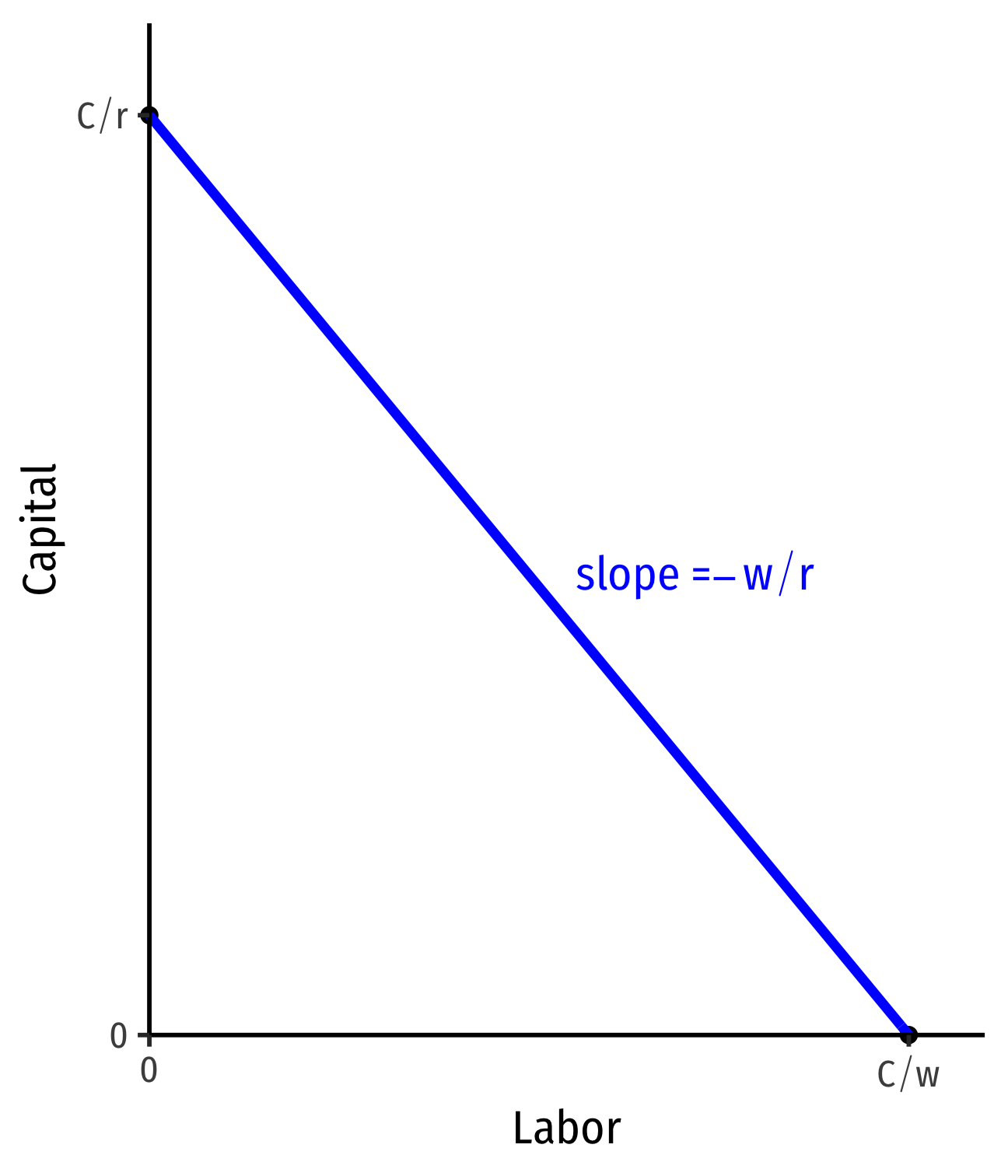
The Isocost Line, Graphically
wl+rk=C
The Isocost Line, Graphically
wl+rk=C
- Solve for k to graph
k=Cr−wrl
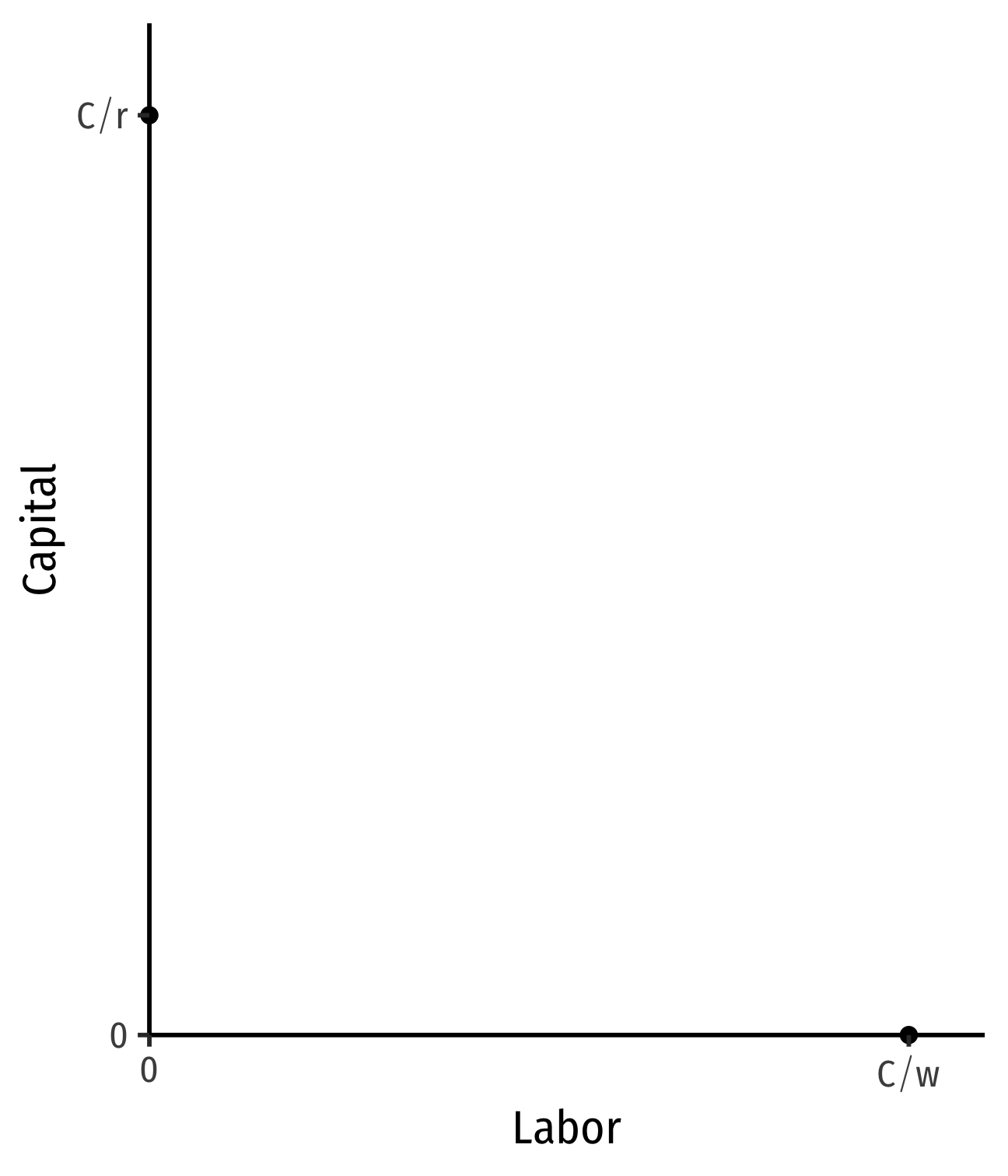
The Isocost Line, Graphically
wl+rk=C
- Solve for k to graph
k=Cr−wrl
- Vertical-intercept: Cr
- Horizontal-intercept: Cw
The Isocost Line, Graphically
wl+rk=C
- Solve for k to graph
k=Cr−wrl
- Vertical-intercept: Cr
- Horizontal-intercept: Cw
- slope: −wr

The Isocost Line: Example
Example: Suppose your firm has a purchasing budget of $50. Market wages are $5/worker-hour and the mark rental rate of capital is $10/machine-hour. Let l be on the horizontal axis and k be on the vertical axis.
Write an equation for the isocost line (in graphable form).
Graph the isocost line.
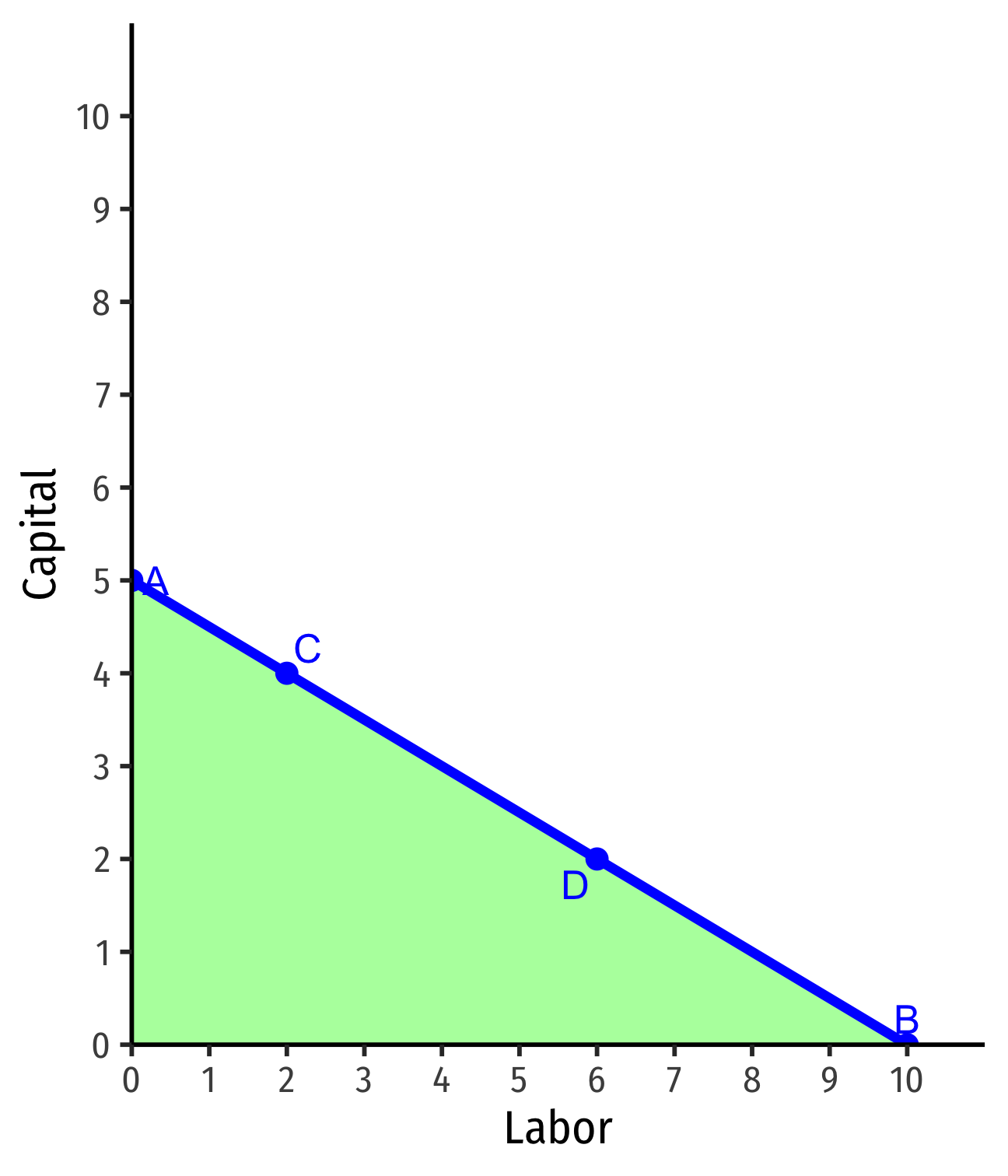
Interpreting the Isocost Line
- Points on the line are same total cost
- A: $5(0l)+$10(5k)=$50
- B: $5(10l)+$10(0k)=$50
- C: $5(2l)+$10(4k)=$50
- D: $5(6l)+$10(2k)=$50
Interpreting the Isocost Line
Points on the line are same total cost
- A: $5(0l)+$10(5k)=$50
- B: $5(10l)+$10(0k)=$50
- C: $5(2l)+$10(4k)=$50
- D: $5(6l)+$10(2k)=$50
Points beneath the line are cheaper (but may produce less)
- E: $5(3l)+$10(2k)=$35
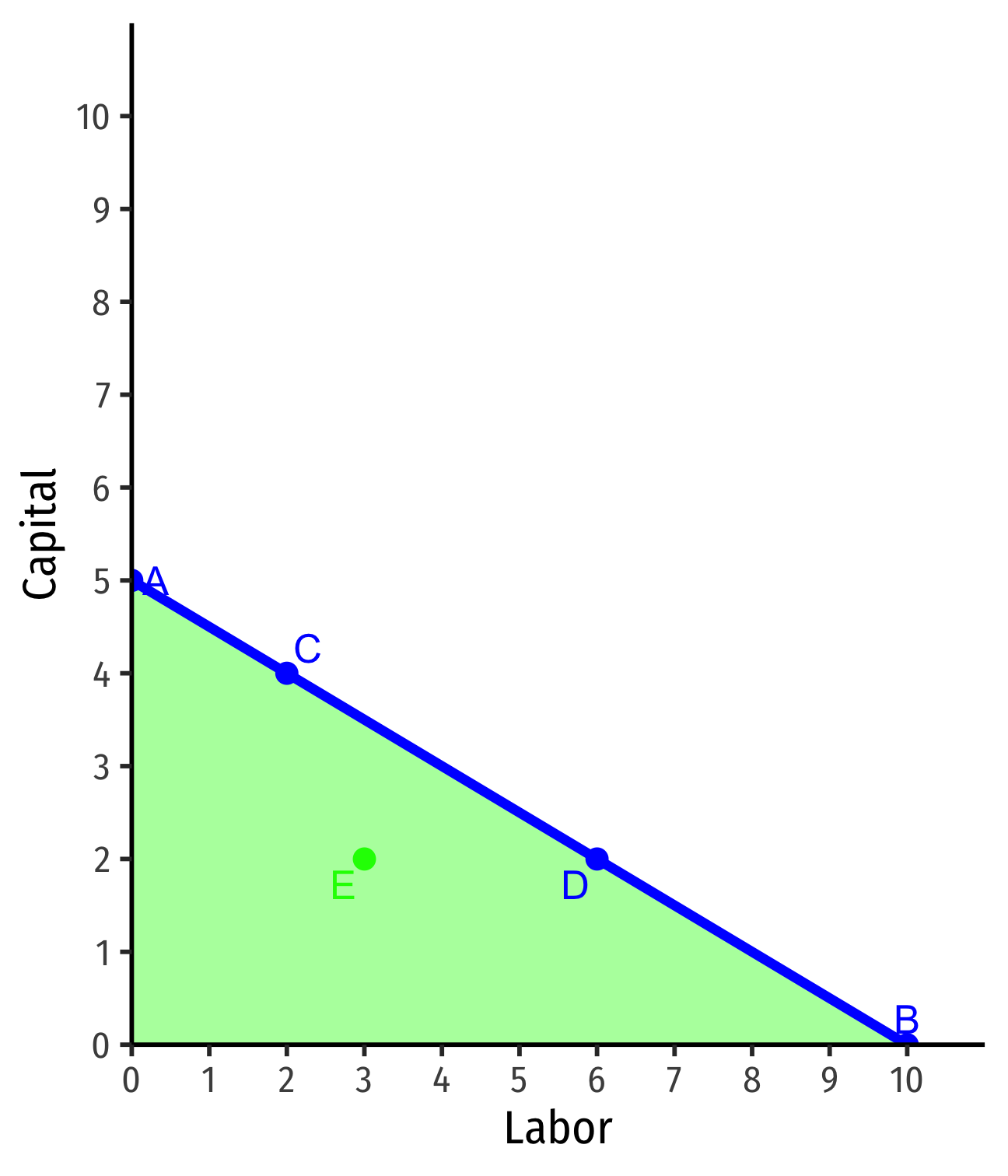
Interpreting the Isocost Line
Points on the line are same total cost
- A: $5(0l)+$10(5k)=$50
- B: $5(10l)+$10(0k)=$50
- C: $5(2l)+$10(4k)=$50
- D: $5(6l)+$10(2k)=$50
Points beneath the line are cheaper (but may produce less)
- E: $5(3l)+$10(2k)=$35
Points above the line are more expensive (and may produce more)
- F: $5(6l)+$10(4k)=$70
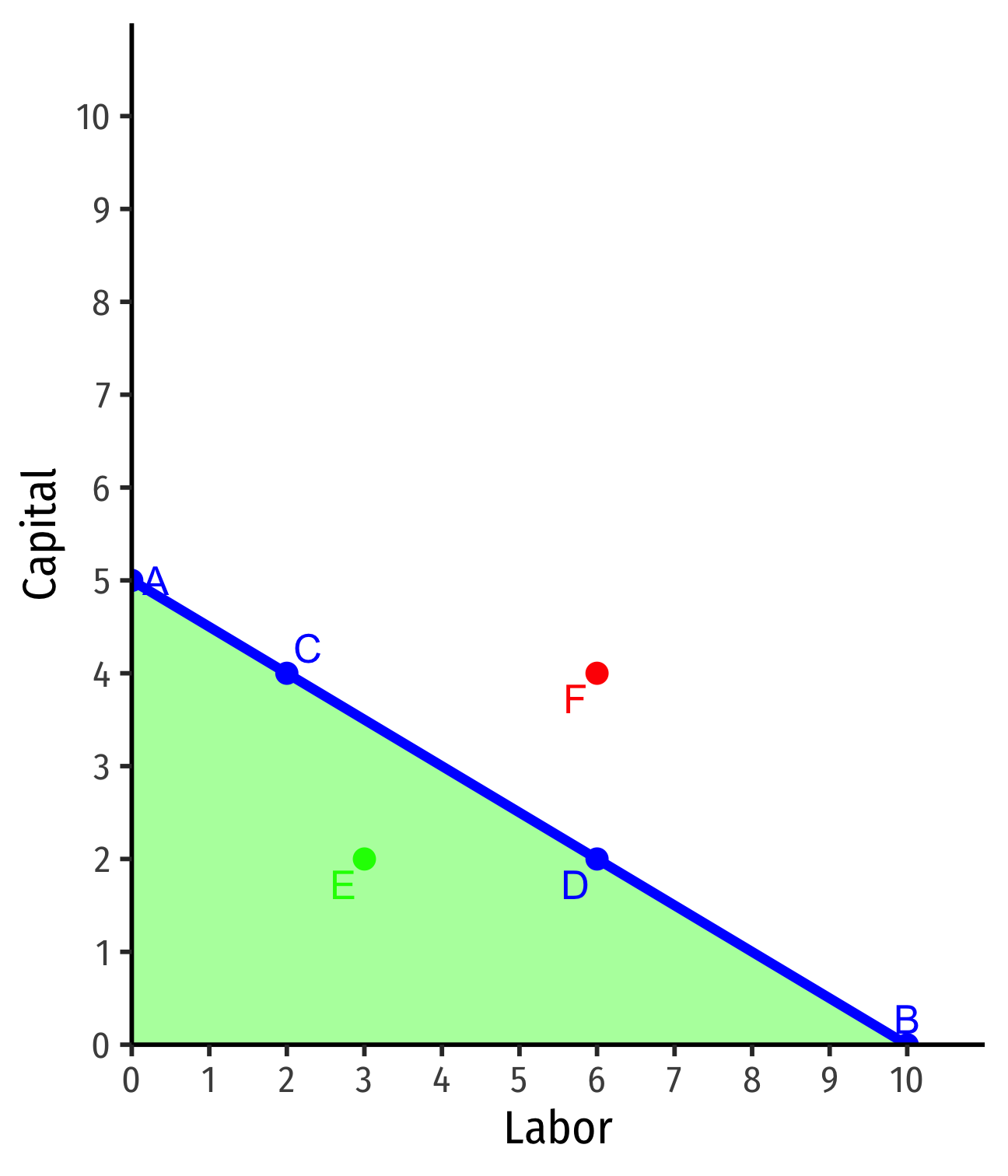
Interpretting the Slope
Slope: tradeoff between l and k at market prices
- Market “exchange rate” between l and k
Relative price of l or the opportunity cost of l:
Hiring 1 more unit of l requires giving up (wr) units of k
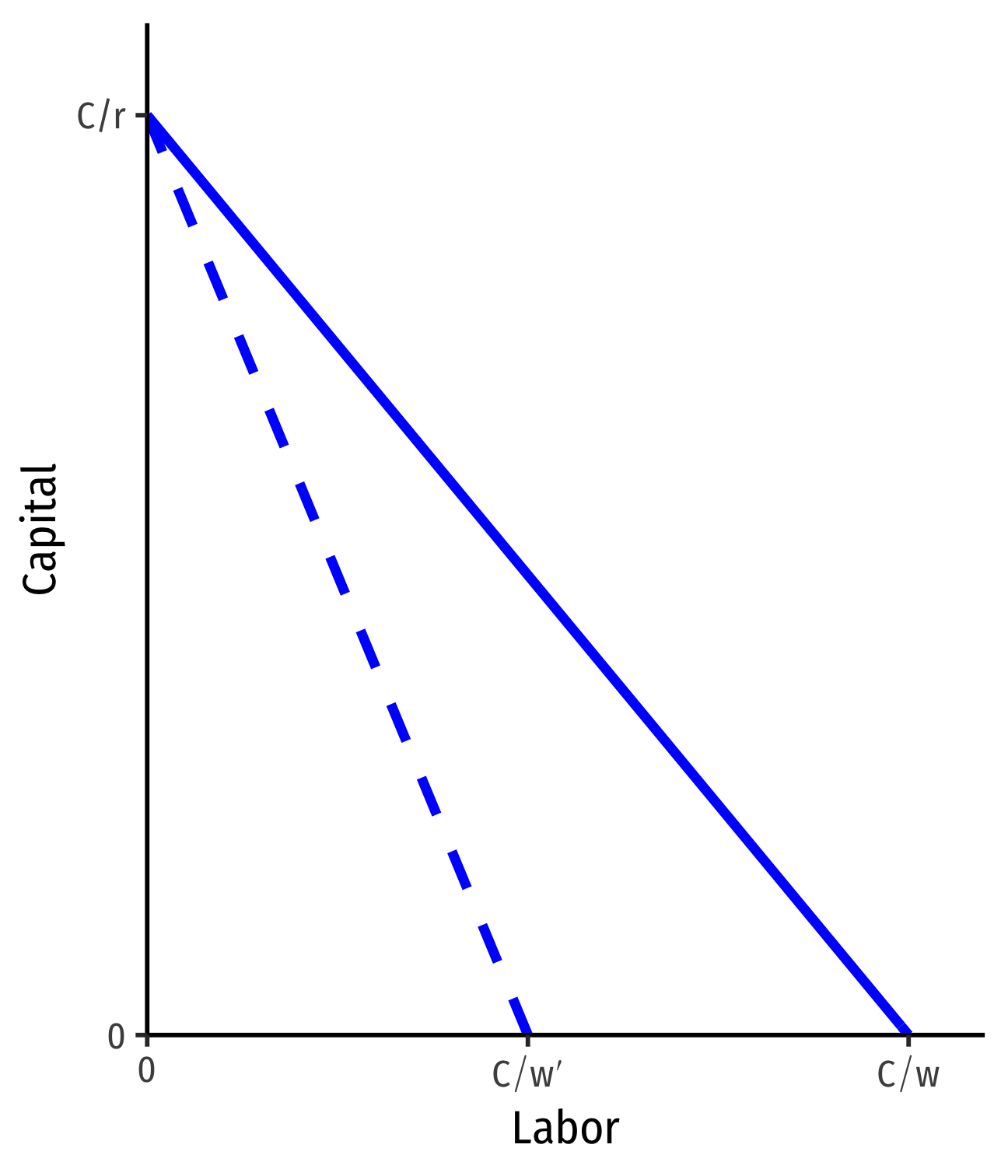
Changes in Relative Factor Prices I
- Changes in relative factor prices: rotate the line
Example: An increase in the price of l
- Slope changes: −w′r

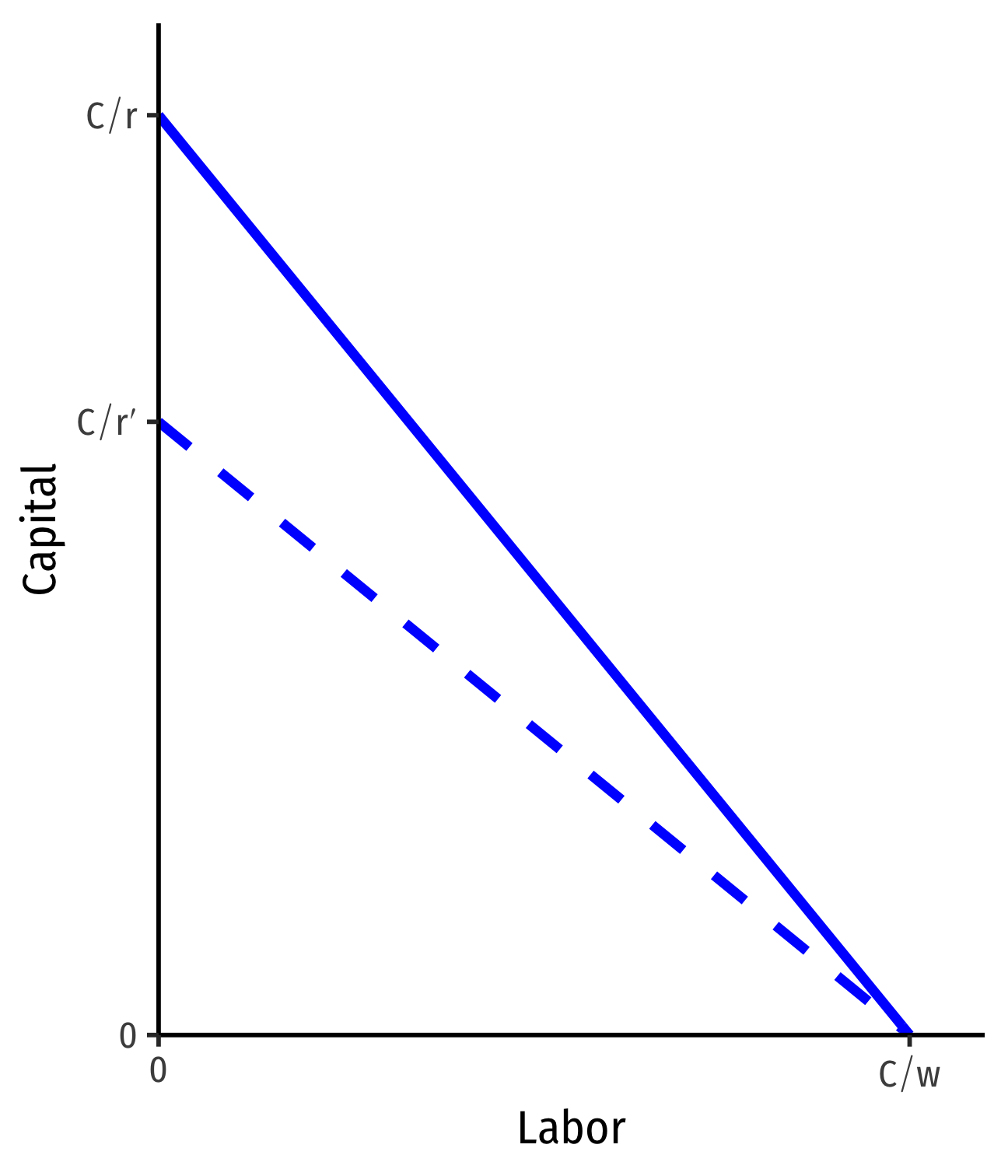
Changes in Relative Factor Prices II
- Changes in relative factor prices: rotate the line
Example: An increase in the price of k
- Slope changes: −wr′