1.4 — Utility Maximization
ECON 306 • Microeconomic Analysis • Spring 2022
Ryan Safner
Assistant Professor of Economics
safner@hood.edu
ryansafner/microS22
microS22.classes.ryansafner.com
Constrained Optimization
Constrained Optimization I
We model most situations as a constrained optimization problem:
People optimize: make tradeoffs to achieve their objective as best as they can
Subject to constraints: limited resources (income, time, attention, etc)

Constrained Optimization II
One of the most generally useful mathematical models
Endless applications: how we model nearly every decision-maker
consumer, business firm, politician, judge, bureaucrat, voter, dictator, pirate, drug cartel, drug addict, parent, child, etc
- Key economic skill: recognizing how to apply the model to a situation

Remember!

Constrained Optimization III
- All constrained optimization models have three moving parts:

Constrained Optimization III
- All constrained optimization models have three moving parts:
- Choose: < some alternative >

Constrained Optimization III
- All constrained optimization models have three moving parts:
Choose: < some alternative >
In order to maximize: < some objective >

Constrained Optimization III
- All constrained optimization models have three moving parts:
Choose: < some alternative >
In order to maximize: < some objective >
Subject to: < some constraints >

Constrained Optimization: Example I
Example: A Hood student picking courses hoping to achieve the highest GPA while getting an Econ major.
Choose:
In order to maximize:
Subject to:

Constrained Optimization: Example II
Example: How should FedEx plan its delivery route?
Choose:
In order to maximize:
Subject to:
Constrained Optimization: Example III
Example: The U.S. government wants to remain economically competitive but reduce emissions by 25%.
Choose:
In order to maximize:
Subject to:

Constrained Optimization: Example IV
Example: How do elected officials make decisions in politics?
Choose:
In order to maximize:
Subject to:

The Utility Maximization Problem
- The individual's utility maximization problem we've been modeling, finally, is:
Choose: < a consumption bundle >
In order to maximize: < utility >
Subject to: < income and market prices >

The Utility Maximization Problem: Tools
We now have the tools to understand individual choices:
Budget constraint: individual’s constraints of income and market prices
- How market trades off between goods
- Marginal cost (of good x, in terms of y)
Utility function: individual’s objective to maximize, based on their preferences
- How individual trades off between goods
- Marginal benefit (of good x, in terms of y)
The Utility Maximization Problem: Verbally
- The individual's constrained optimization problem:
choose a bundle of goods to maximize utility, subject to income and market prices
The Utility Maximization Problem: Mathematically
maxx,y≥0u(x,y) s.t.pxx+pyy=m
- This requires calculus to solve.† We will look at graphs instead!

† See the mathematical appendix in today's class notes on how to solve it with calculus, and an example.
The Individual's Optimum: Graphically
- Graphical solution: Highest indifference curve tangent to budget constraint
- Bundle A!

The Individual's Optimum: Graphically
Graphical solution: Highest indifference curve tangent to budget constraint
- Bundle A!
B or C spend all income, but a better combination exists
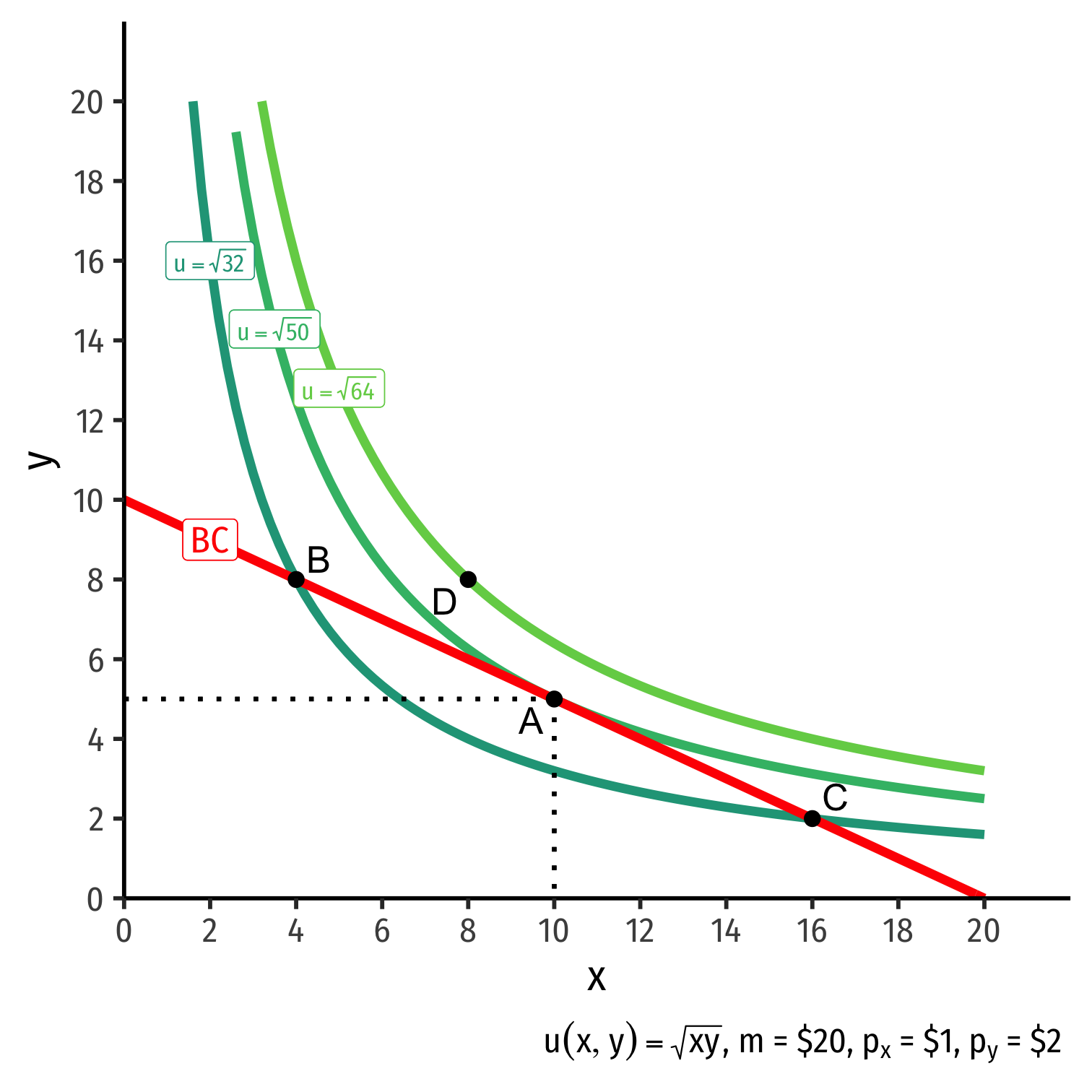
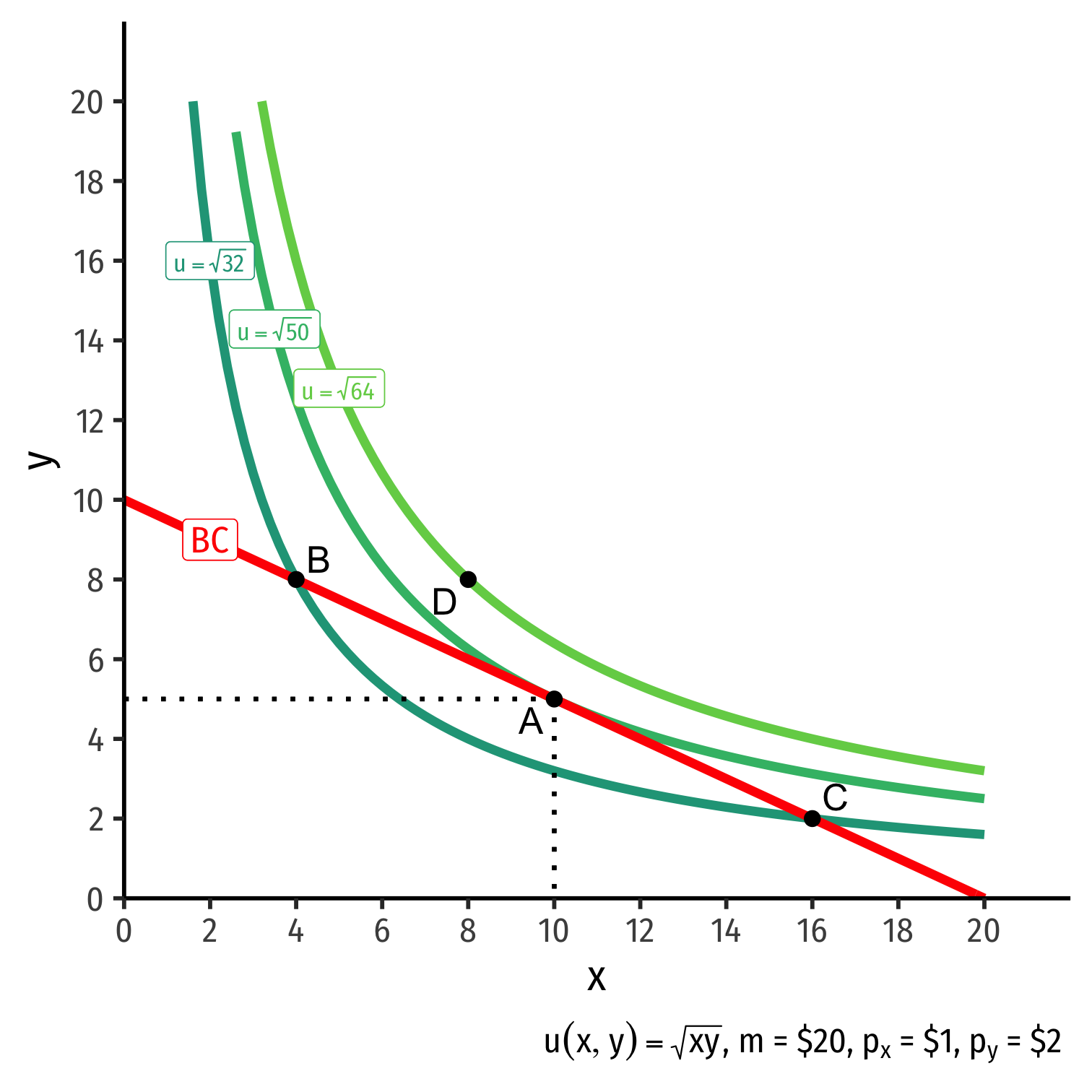
The Individual's Optimum: Graphically
Graphical solution: Highest indifference curve tangent to budget constraint
- Bundle A!
B or C spend all income, but a better combination exists
D is higher utility, but not affordable at current income & prices
The Individual's Optimum: Why Not B?
indiff. curve slope>budget constr. slope
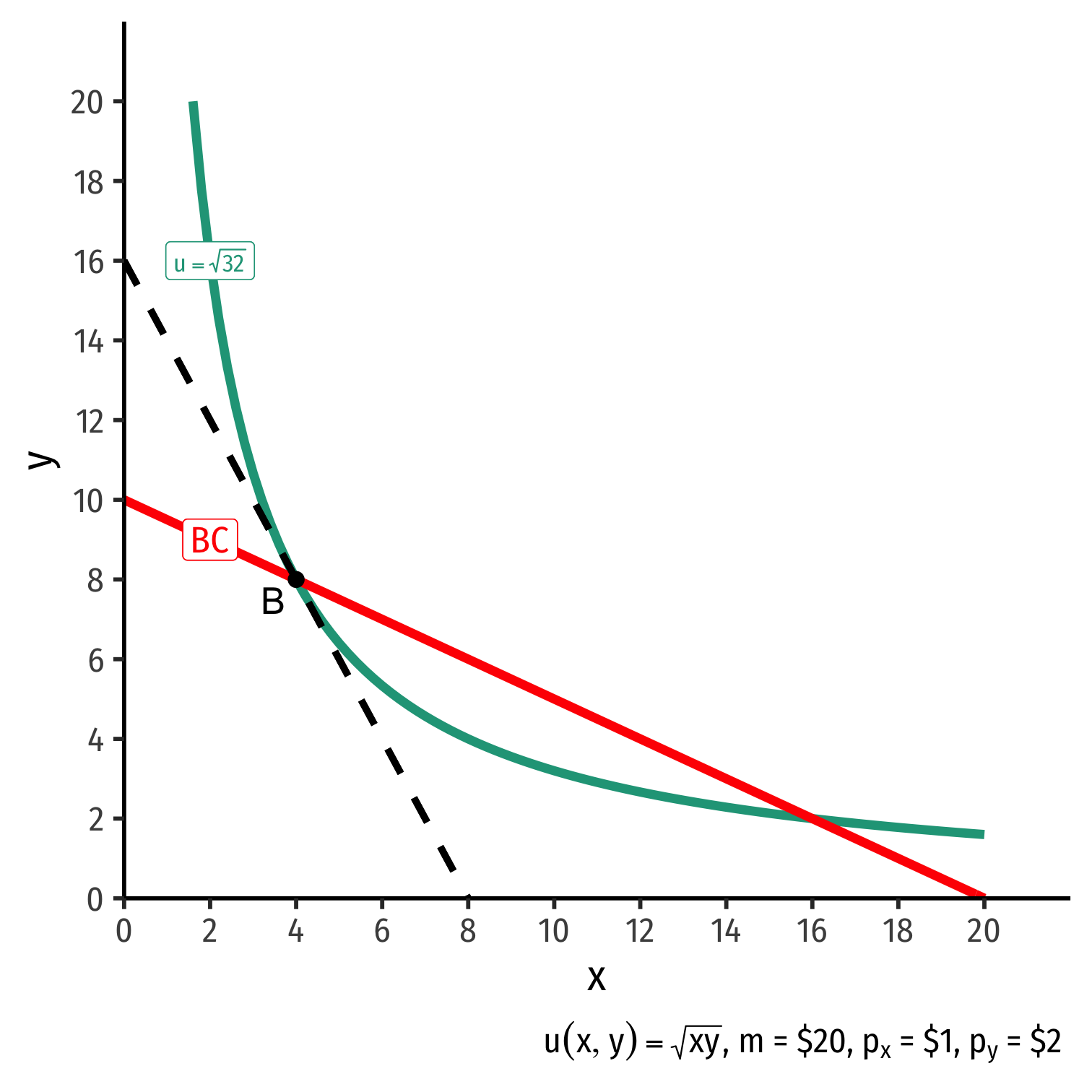
The Individual's Optimum: Why Not B?
indiff. curve slope>budget constr. slopeMUxMUy>pxpy2>0.5
Consumer views MB of x is 2 units of y
- Consumer’s “exchange rate:” 2Y:1X
Market-determined MC of x is 0.5 units of y
- Market exchange rate is 0.5Y:1X
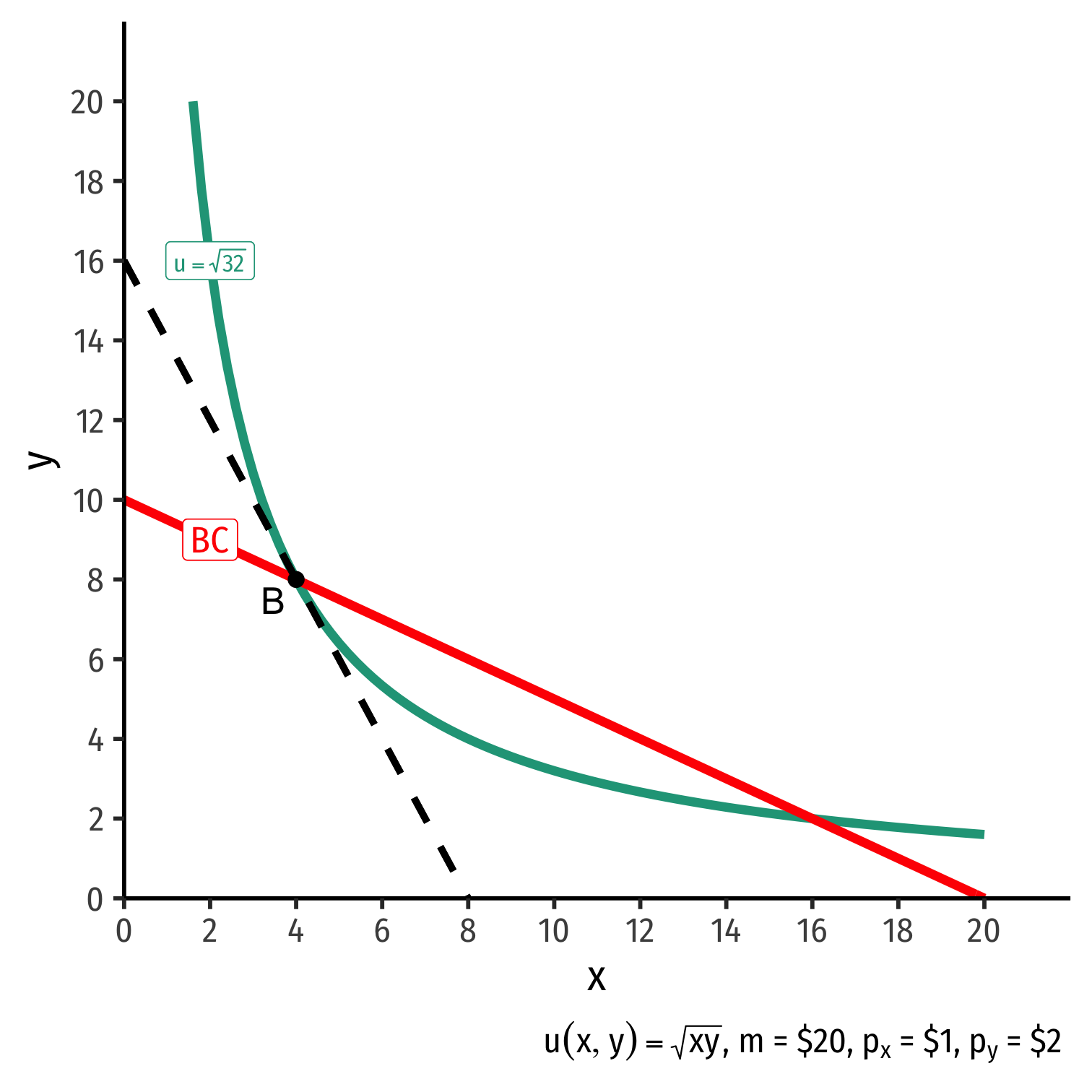
The Individual's Optimum: Why Not B?
indiff. curve slope>budget constr. slopeMUxMUy>pxpy2>0.5
Consumer views MB of x is 2 units of y
- Consumer’s “exchange rate:” 2Y:1X
Market-determined MC of x is 0.5 units of y
- Market exchange rate is 0.5Y:1X
Can spend less on y, more on x for more utility!

The Individual's Optimum: Why Not C?
indiff. curve slope<budget constr. slope
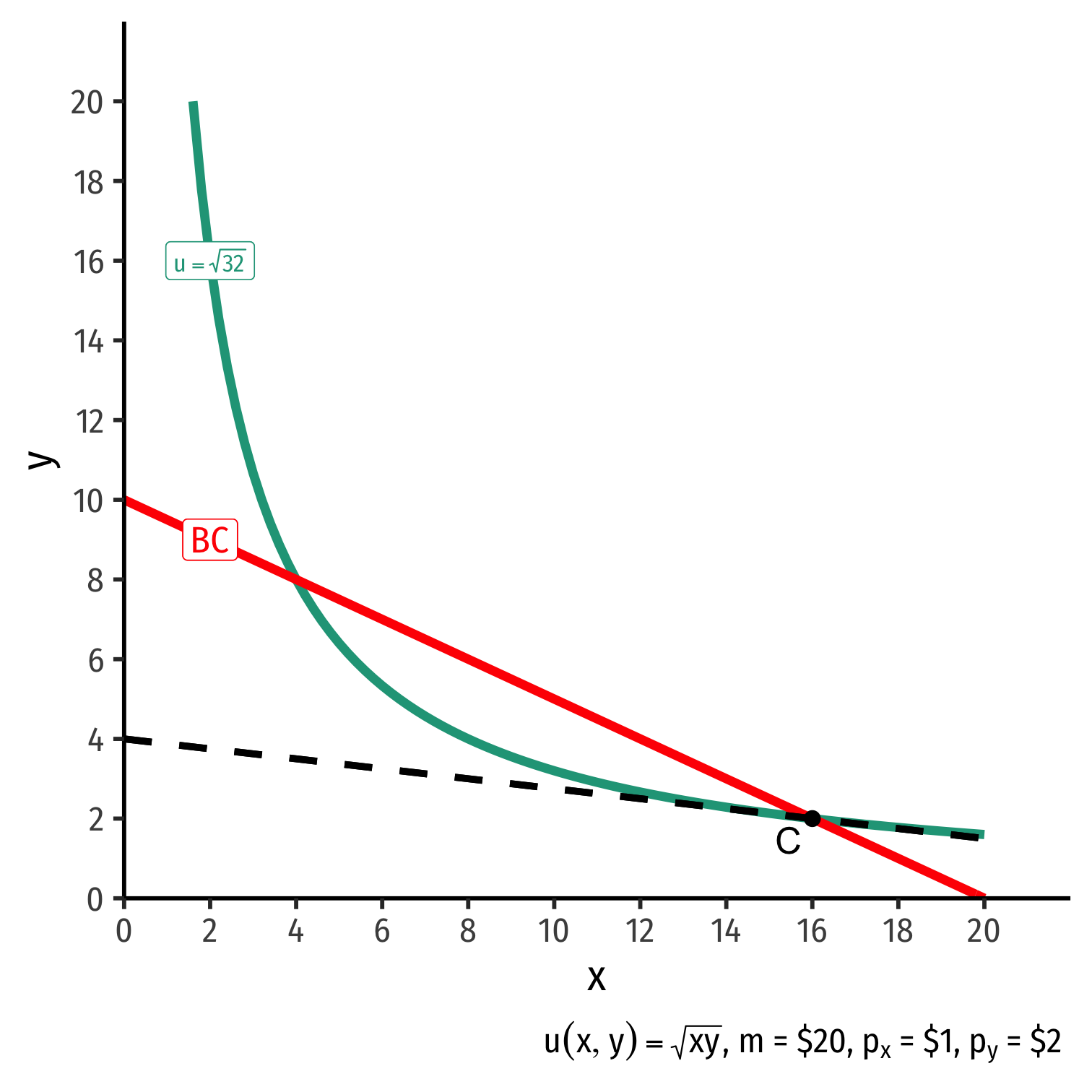
The Individual's Optimum: Why Not C?
indiff. curve slope<budget constr. slopeMUxMUy<pxpy0.125<0.5
Consumer views MB of x is 0.125 units of y
- Consumer’s “exchange rate:” 0.125Y:1X
Market-determined MC of x is 0.5 units of y
- Market exchange rate is 0.5Y:1X
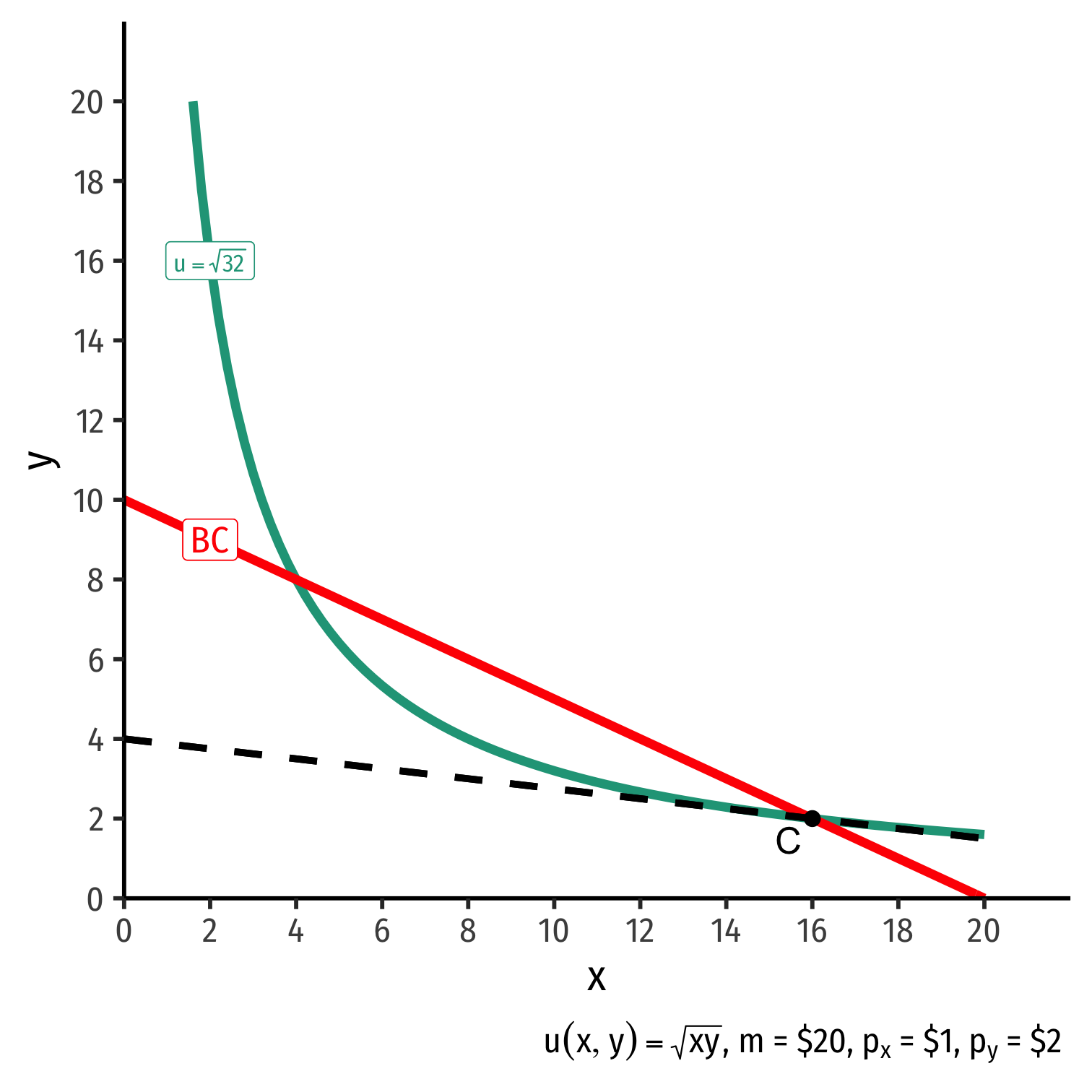
The Individual's Optimum: Why Not C?
indiff. curve slope<budget constr. slopeMUxMUy<pxpy0.125<0.5
Consumer views MB of x is 0.125 units of y
- Consumer’s “exchange rate:” 0.125Y:1X
Market-determined MC of x is 0.5 units of y
- Market exchange rate is 0.5Y:1X
Can spend less on y, more on x for more utility!
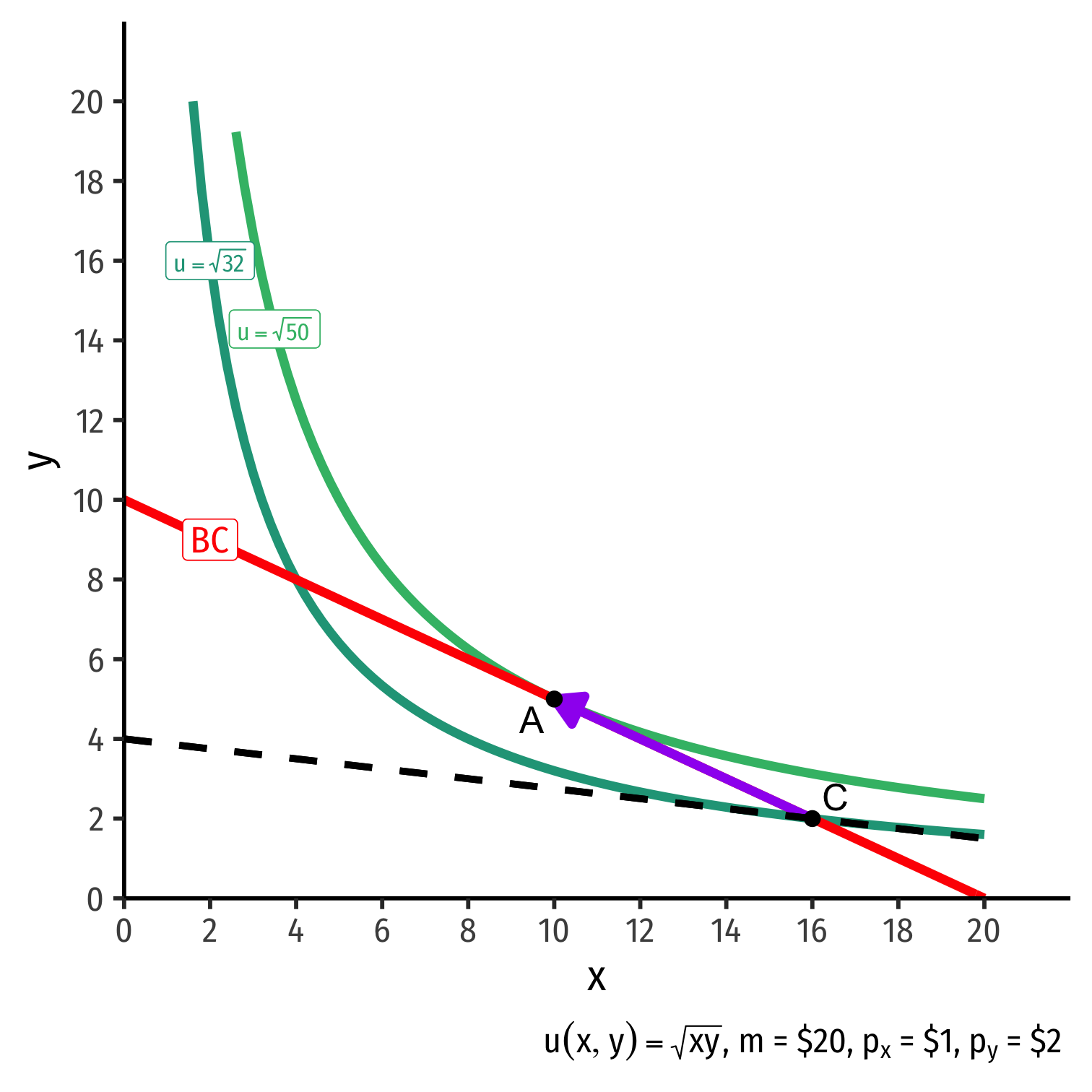
The Individual's Optimum: Why A?
indiff. curve slope=budget constr. slope
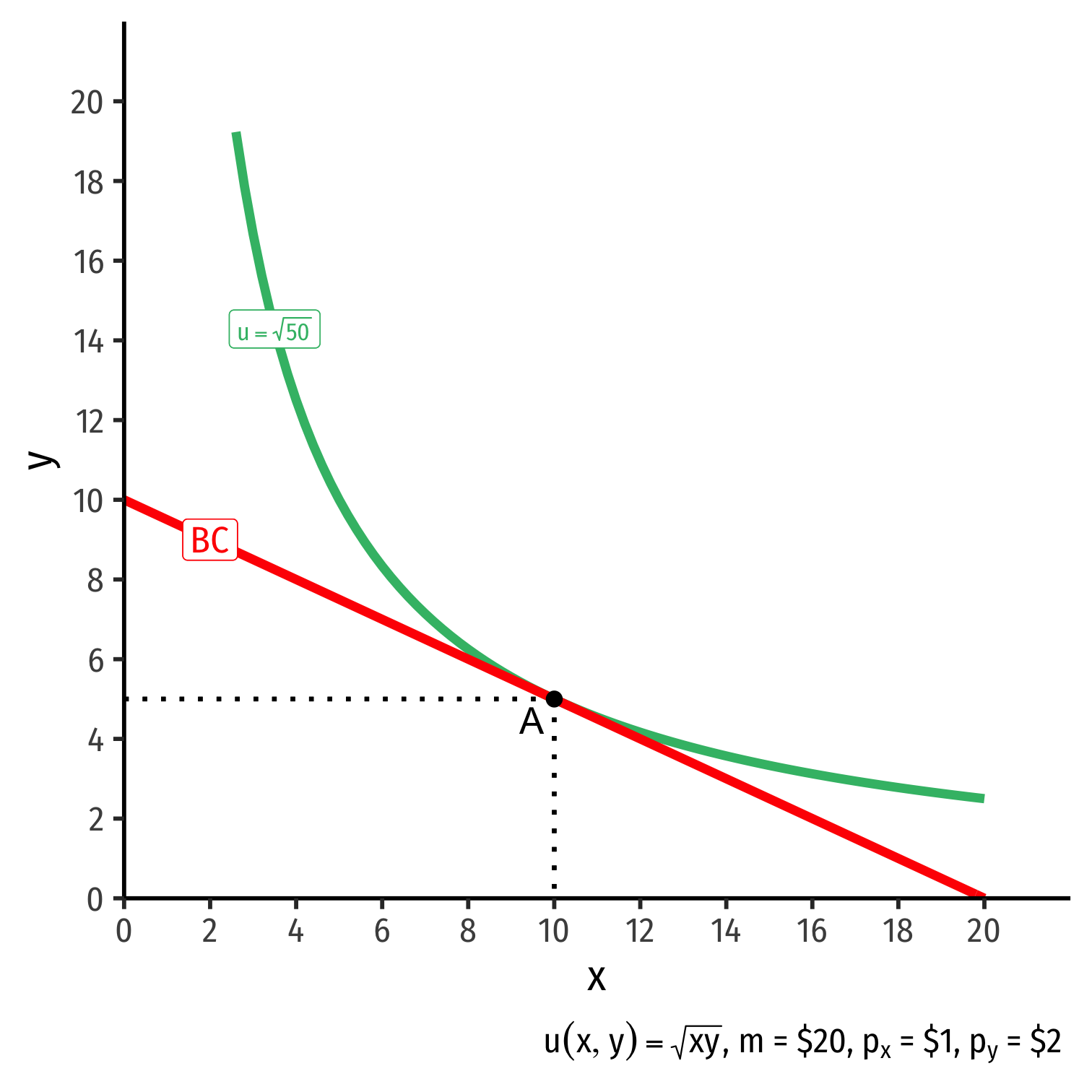
The Individual's Optimum: Why A?
indiff. curve slope=budget constr. slopeMUxMUy=pxpy0.5=0.5
Marginal benefit = Marginal cost
- Consumer exchanges at same rate as market
No other combination of (x,y) exists that could increase utility!†
† At current income and market prices!
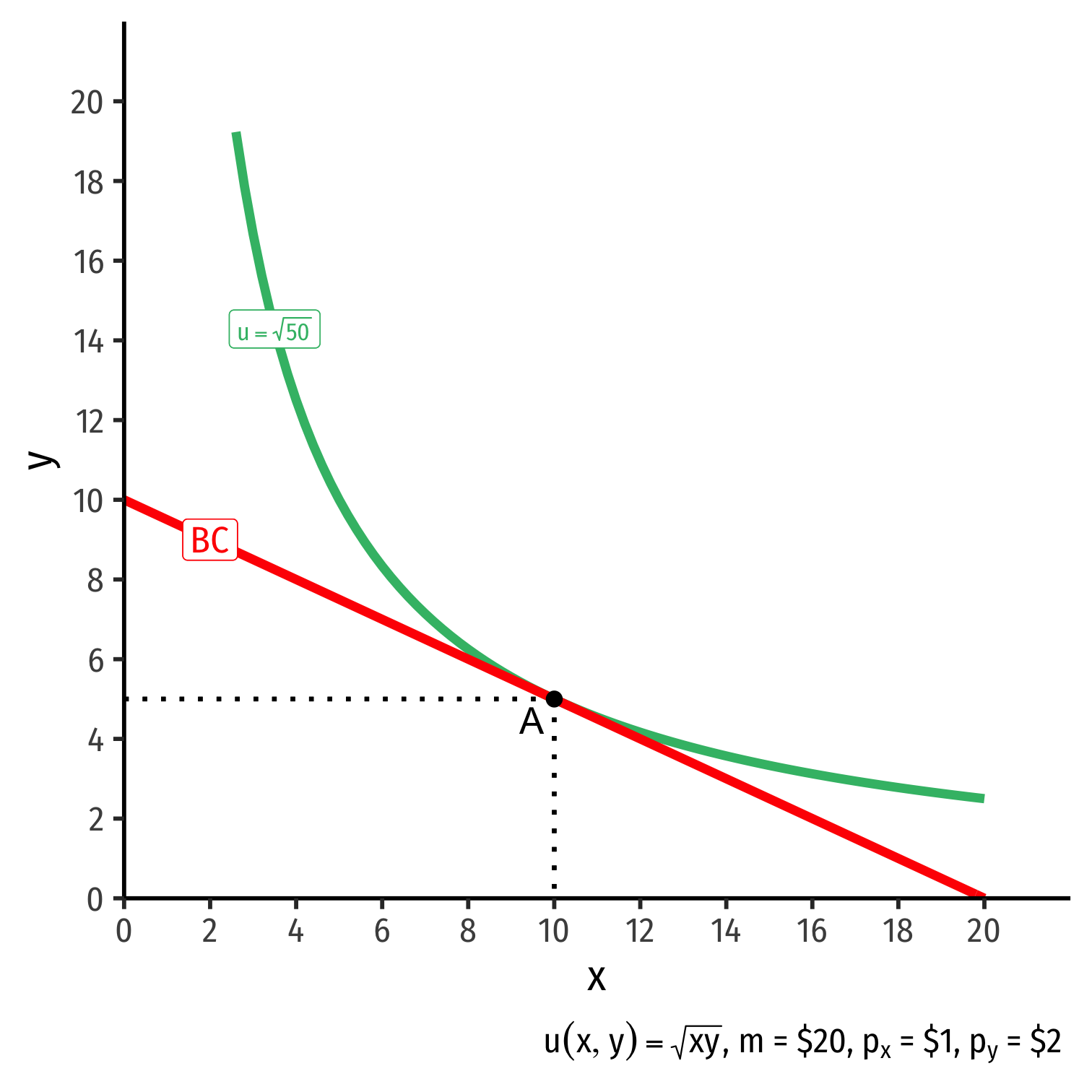
The Individual's Optimum: Two Equivalent Rules
Rule 1
MUxMUy=pxpy
- Easier for calculation (slopes)
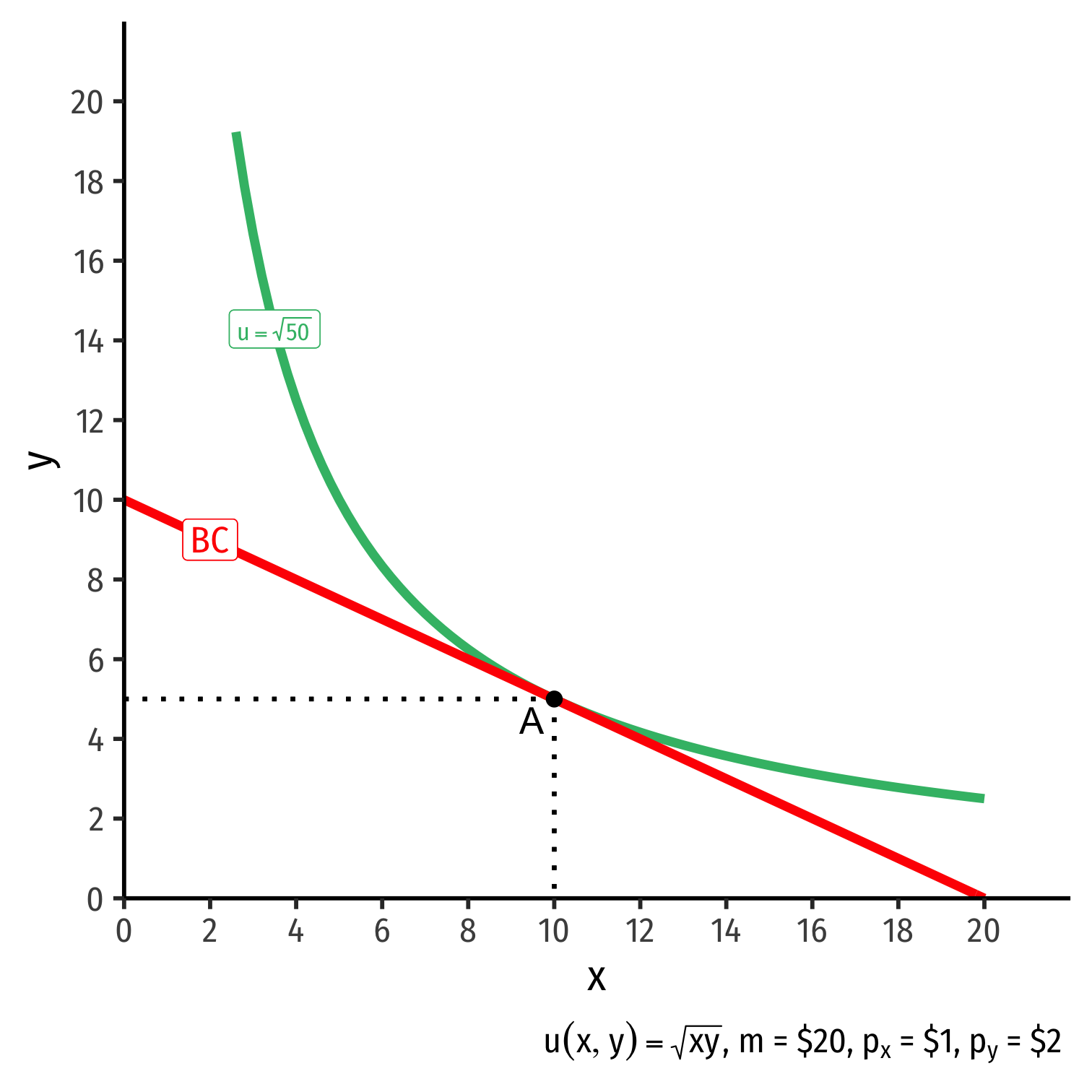
The Individual's Optimum: Two Equivalent Rules
Rule 1
MUxMUy=pxpy
- Easier for calculation (slopes)
Rule 2
MUxpx=MUypy
- Easier for intuition (next slide)
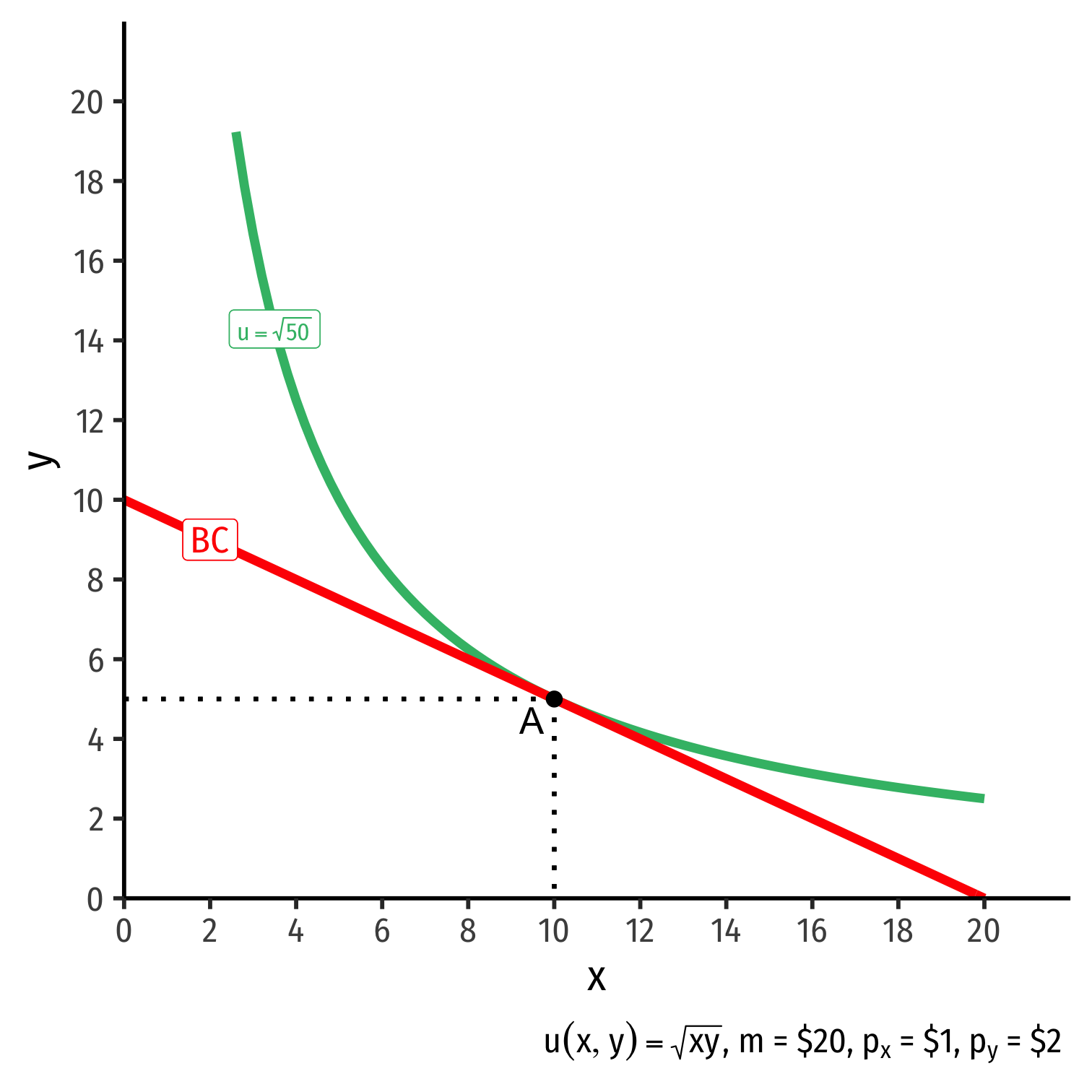
The Individual's Optimum: The Equimarginal Rule
MUxpx=MUypy=⋯=MUnpn
Equimarginal Rule: consumption is optimized where the marginal utility per dollar spent is equalized across all n possible goods/decisions
Always choose an option that gives higher marginal utility (e.g. if MUx<MUy), consume more y!
- But each option has a different price, so weight each option by its price, hence MUxpx
An Optimum, By Definition
Any optimum in economics: no better alternatives exist under current constraints
No possible change in your consumption that would increase your utility

Practice I
Example: You can get utility from consuming bags of Almonds (a) and bunches of Bananas (b), according to the utility function:
u(a,b)=abMUa=bMUb=a
You have an income of $50, the price of Almonds is $10, and the price of Bananas is $2. Put Almonds on the horizontal axis and Bananas on the vertical axis.
- What is your utility-maximizing bundle of Almonds and Bananas?
- How much utility does this provide? [Does the answer to this matter?]
Practice II, Cobb-Douglas!
Example: You can get utility from consuming Burgers (b) and Fries (f), according to the utility function:
u(b,f)=√bfMUb=0.5b−0.5f0.5MUf=0.5b0.5f−0.5
You have an income of $20, the price of Burgers is $5, and the price of Fries is $2. Put Burgers on the horizontal axis and Fries on the vertical axis.
- What is your utility-maximizing bundle of Burgers and Fries?
- How much utility does this provide?
